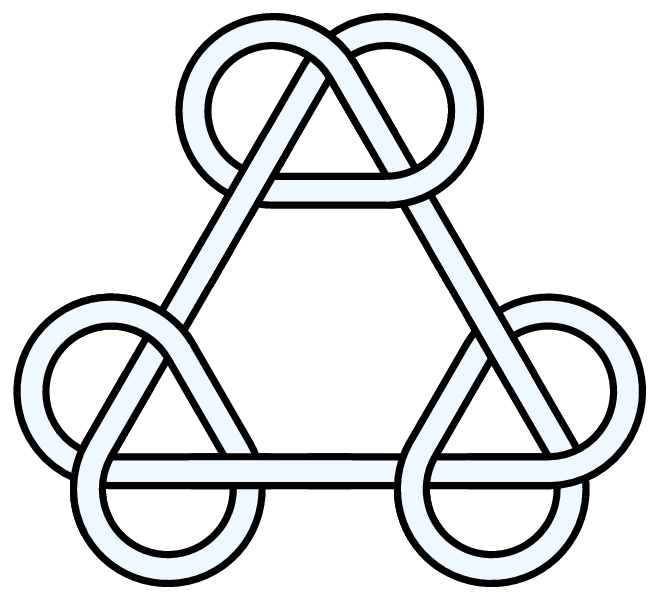
|
|
| Line 17: |
Line 17: |
|
}} |
|
}} |
|
{{Knot View Template| |
|
{{Knot View Template| |
|
image = DylansTrefoil_120.jpg | |
|
image = Blue Trefoil Knot.png | |
|
⚫ |
|
| ⚫ |
text = Thurston's Trefoil - Figure Eight Trick [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/TrefoilFigureEight/index.html]| |
|
|
}} |
|
}} |
|
⚫ |
|
|
|
|
|
| ⚫ |
|
|
|
<div class="NavFrame"><div class="NavHead">Further images...</div> |
|
<div class="NavFrame"><div class="NavHead">Further images...</div> |
|
<div class="NavContent"> |
|
<div class="NavContent"> |
|
{| style="background: transparent;" |
|
{| style="background: transparent;" |
|
⚫ |
|
|
⚫ |
|
|
⚫ |
image = Trefoil-triquetra-circular-arcs-around-triangle.png | |
|
⚫ |
text = Ribbons (straight lines and 2/3 circles)| |
|
⚫ |
|
|
⚫ |
|
|
⚫ |
image = Triquetra-Vesica.png | |
|
⚫ |
text = Ribbons (circular arcs)| |
|
⚫ |
|
|
⚫ |
|
|
⚫ |
image = Triquetra-tightly-knotted.png | |
|
⚫ |
text = Tightly-knotted form| |
|
⚫ |
|
|
⚫ |
|
|
⚫ |
image = Vodicka triquetra.png | |
|
|
text = Celtic| |
|
⚫ |
|
|
|- valign=top |
|
|- valign=top |
|
{{Knot View Template| |
|
{{Knot View Template| |
| Line 39: |
Line 56: |
|
}} |
|
}} |
|
{{Knot View Template| |
|
{{Knot View Template| |
|
image = Auryn_120.gif | |
|
image = DylansTrefoil_120.jpg | |
|
⚫ |
text = Thurston's Trefoil - Figure Eight Trick [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/TrefoilFigureEight/index.html]| |
| ⚫ |
text = The NeverEnding Story is a connected sum of two trefoils. [http://www.thealmightyguru.com/Reviews/NeverendingStory/NeverendingStory.html]| |
|
|
}} |
|
}} |
|
|- valign=top |
|
|- valign=top |
| Line 58: |
Line 75: |
|
image = KenyanStone_160.jpg | |
|
image = KenyanStone_160.jpg | |
|
text = A Kenyan Stone [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/KenyanStone.html]| |
|
text = A Kenyan Stone [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/KenyanStone.html]| |
| ⚫ |
|
|
| ⚫ |
|
|
| ⚫ |
|
|
| ⚫ |
image = Trefoil-triquetra-circular-arcs-around-triangle.png | |
|
| ⚫ |
text = Ribbons (straight lines and 2/3 circles)| |
|
| ⚫ |
|
|
| ⚫ |
|
|
| ⚫ |
image = Triquetra-Vesica.png | |
|
| ⚫ |
text = Ribbons (circular arcs)| |
|
| ⚫ |
|
|
| ⚫ |
|
|
| ⚫ |
image = Triquetra-tightly-knotted.png | |
|
| ⚫ |
text = Tightly-knotted form| |
|
| ⚫ |
|
|
| ⚫ |
|
|
| ⚫ |
image = Vodicka triquetra.png | |
|
| ⚫ |
|
|
|
}} |
|
}} |
|
|- valign=top |
|
|- valign=top |
| Line 92: |
Line 92: |
|
image = RopeTrick_160.jpg | |
|
image = RopeTrick_160.jpg | |
|
text = Mike Hutchings' Rope Trick [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/RopeTrick/index.html]| |
|
text = Mike Hutchings' Rope Trick [http://www.math.toronto.edu/~drorbn/Gallery/KnottedObjects/RopeTrick/index.html]| |
|
|
}} |
|
|
|- valign=top |
|
|
{{Knot View Template| |
|
|
image = Auryn_120.gif | |
|
⚫ |
text = The NeverEnding Story logo is a connected sum of two trefoils. [http://www.thealmightyguru.com/Reviews/NeverendingStory/NeverendingStory.html]| |
|
}} |
|
}} |
|
|} |
|
|} |
Revision as of 23:47, 22 March 2010
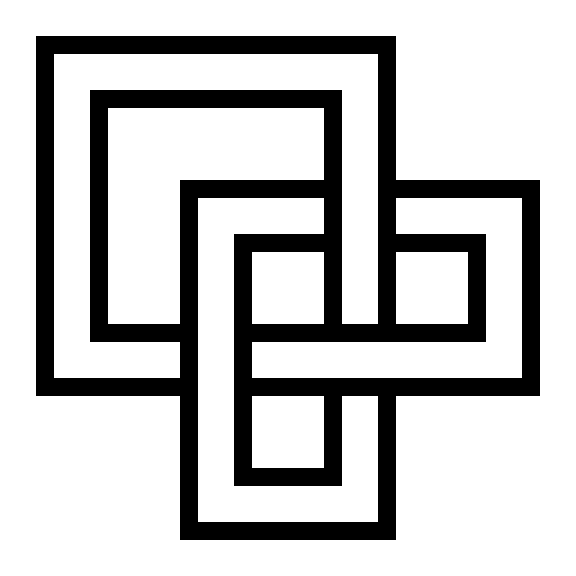
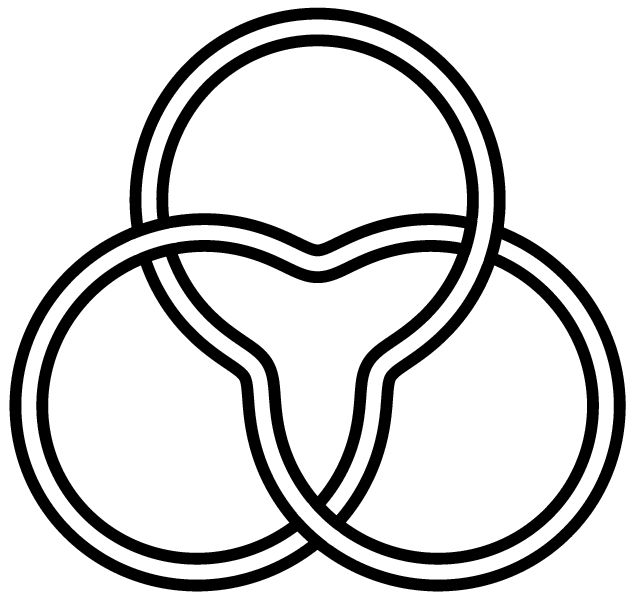
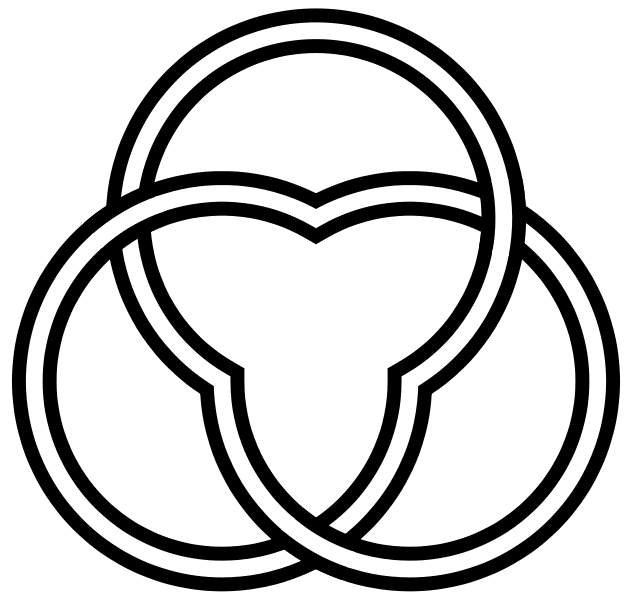
The trefoil is perhaps the easiest knot to find in "nature", and is topologically equivalent to the interlaced form of the common Christian and pagan "triquetra" symbol [12]:
 Logo of Caixa Geral de Depositos, Lisboa [1] |
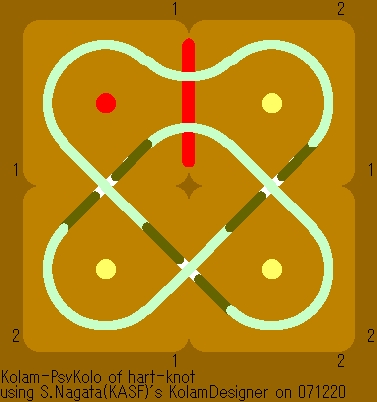 A knot consists of two harts in Kolam [2] |
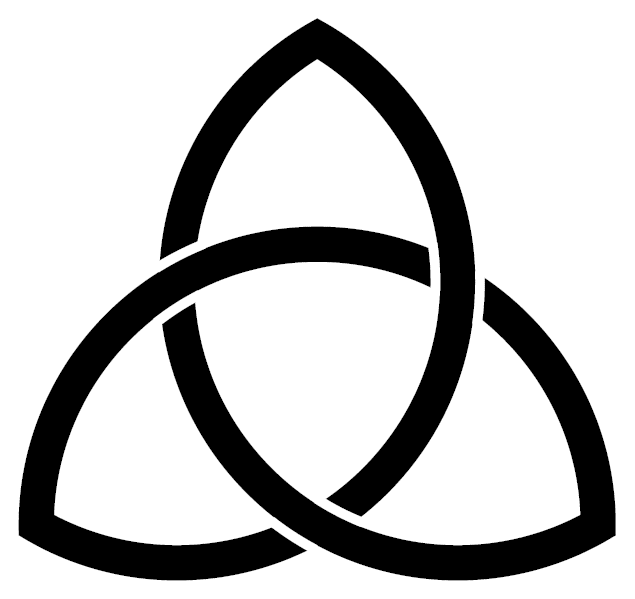 A basic form of the interlaced Triquetra; as a Christian symbol, it refers to the Trinity |
|
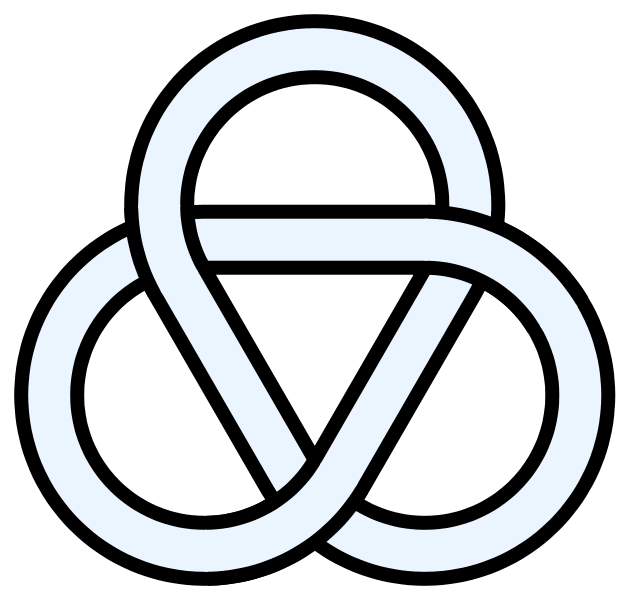 Ribbons (straight lines and 2/3 circles) |
|
|
|
|
|
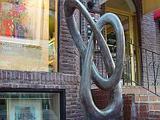 A trefoil near the Hollander York Gallery [4] |
|
 Thurston's Trefoil - Figure Eight Trick [6] |
|
|
 A hagfish tying itself in a knot to escape capture. [8] |
 One version of the Germanic "Valknut" symbol |
|
|
|
 In the form of an architectural trefoil |
|
 Mike Hutchings' Rope Trick [10] |
 The NeverEnding Story logo is a connected sum of two trefoils. [11] |
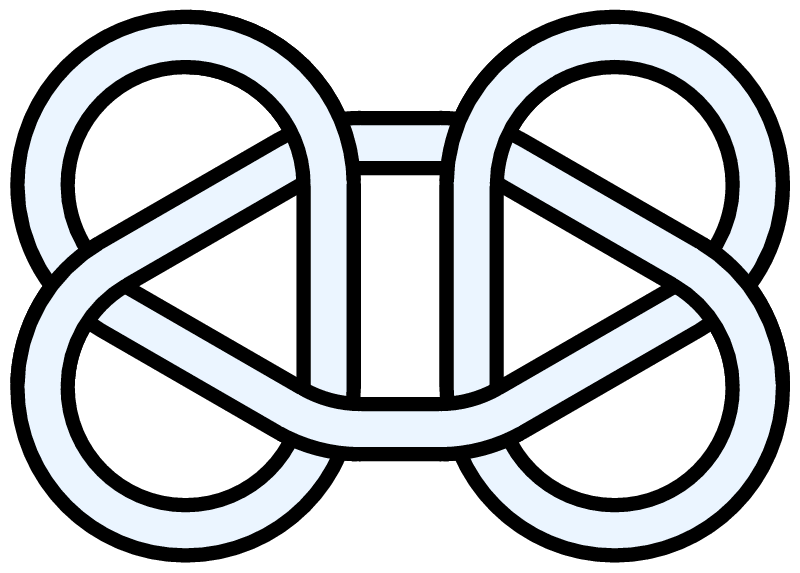
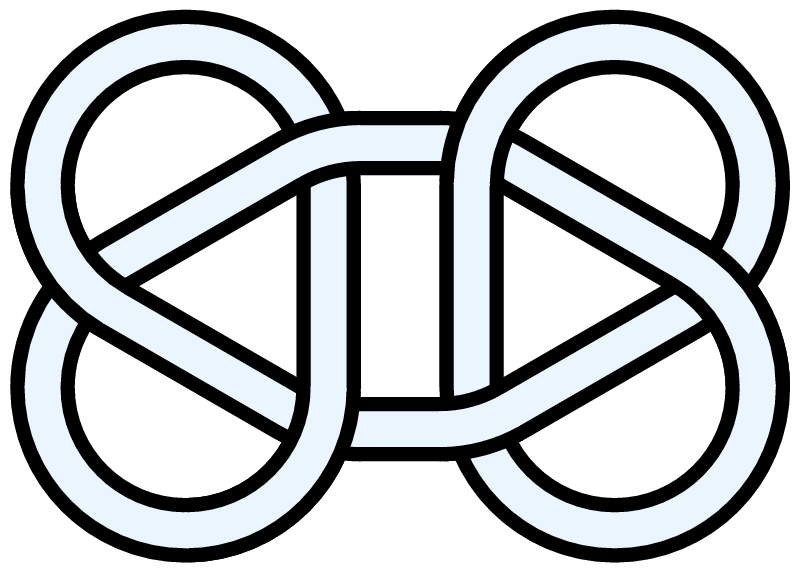
Non-prime (compound) versions
Two trefoils (single-closed-loop version of the "square knot" of practical knot-tying)
Two trefoils (single-closed-loop version of the "granny knot" of practical knot-tying).
Three trefoils (symmetrical).
Four trefoils (Celtic or pseudo-Celtic decorative knot which fits in square)
Three trefoils along a closed loop which itself is knotted as a trefoil.
For a configuration of two trefoils along a closed loop which is prime, see 10_120.