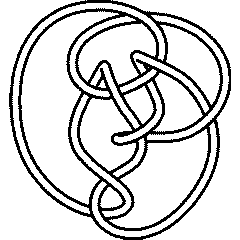10 112: Difference between revisions
No edit summary |
No edit summary |
||
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
<!-- WARNING! WARNING! WARNING! |
|||
<!-- This page was generated from the splice base [[Rolfsen_Splice_Base]]. Please do not edit! |
|||
<!-- You probably want to edit the template referred to immediately below. (See [[Category:Knot Page Template]].) |
|||
<!-- This page itself was created by running [[Media:KnotPageSpliceRobot.nb]] on [[Rolfsen_Splice_Base]]. --> |
|||
<!-- --> |
<!-- --> |
||
<!-- --> |
|||
<!-- --> |
<!-- --> |
||
{{Rolfsen Knot Page| |
|||
<!-- --> |
|||
n = 10 | |
|||
<!-- provide an anchor so we can return to the top of the page --> |
|||
k = 112 | |
|||
<span id="top"></span> |
|||
KnotilusURL = http://srankin.math.uwo.ca/cgi-bin/retrieve.cgi/1,-5,2,-6,8,-1,9,-2,10,-8,3,-7,4,-9,5,-10,6,-3,7,-4/goTop.html | |
|||
<!-- --> |
|||
braid_table = <table cellspacing=0 cellpadding=0 border=0> |
|||
<!-- this relies on transclusion for next and previous links --> |
|||
<tr><td>[[Image:BraidPart3.gif]][[Image:BraidPart3.gif]][[Image:BraidPart3.gif]][[Image:BraidPart0.gif]][[Image:BraidPart3.gif]][[Image:BraidPart0.gif]][[Image:BraidPart3.gif]][[Image:BraidPart0.gif]][[Image:BraidPart3.gif]][[Image:BraidPart0.gif]]</td></tr> |
|||
{{Knot Navigation Links|ext=gif}} |
|||
<tr><td>[[Image:BraidPart4.gif]][[Image:BraidPart4.gif]][[Image:BraidPart4.gif]][[Image:BraidPart1.gif]][[Image:BraidPart4.gif]][[Image:BraidPart1.gif]][[Image:BraidPart4.gif]][[Image:BraidPart1.gif]][[Image:BraidPart4.gif]][[Image:BraidPart1.gif]]</td></tr> |
|||
<tr><td>[[Image:BraidPart0.gif]][[Image:BraidPart0.gif]][[Image:BraidPart0.gif]][[Image:BraidPart2.gif]][[Image:BraidPart0.gif]][[Image:BraidPart2.gif]][[Image:BraidPart0.gif]][[Image:BraidPart2.gif]][[Image:BraidPart0.gif]][[Image:BraidPart2.gif]]</td></tr> |
|||
{{Rolfsen Knot Page Header|n=10|k=112|KnotilusURL=http://srankin.math.uwo.ca/cgi-bin/retrieve.cgi/1,-5,2,-6,8,-1,9,-2,10,-8,3,-7,4,-9,5,-10,6,-3,7,-4/goTop.html}} |
|||
</table> | |
|||
braid_crossings = 10 | |
|||
<br style="clear:both" /> |
|||
braid_width = 3 | |
|||
braid_index = 3 | |
|||
{{:{{PAGENAME}} Further Notes and Views}} |
|||
same_alexander = [[K11a184]], | |
|||
same_jones = | |
|||
{{Knot Presentations}} |
|||
khovanov_table = <table border=1> |
|||
{{3D Invariants}} |
|||
{{4D Invariants}} |
|||
{{Polynomial Invariants}} |
|||
{{Vassiliev Invariants}} |
|||
{{Khovanov Homology|table=<table border=1> |
|||
<tr align=center> |
<tr align=center> |
||
<td width=13.3333%><table cellpadding=0 cellspacing=0> |
<td width=13.3333%><table cellpadding=0 cellspacing=0> |
||
<tr><td>\</td><td> </td><td>r</td></tr> |
|||
<tr><td> </td><td> \ </td><td> </td></tr> |
<tr><td> </td><td> \ </td><td> </td></tr> |
||
<tr><td>j</td><td> </td><td>\</td></tr> |
<tr><td>j</td><td> </td><td>\</td></tr> |
||
</table></td> |
</table></td> |
||
<td width=6.66667%>-6</td ><td width=6.66667%>-5</td ><td width=6.66667%>-4</td ><td width=6.66667%>-3</td ><td width=6.66667%>-2</td ><td width=6.66667%>-1</td ><td width=6.66667%>0</td ><td width=6.66667%>1</td ><td width=6.66667%>2</td ><td width=6.66667%>3</td ><td width=6.66667%>4</td ><td width=13.3333%>χ</td></tr> |
|||
<tr align=center><td>7</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td bgcolor=yellow>1</td><td>1</td></tr> |
<tr align=center><td>7</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td bgcolor=yellow>1</td><td>1</td></tr> |
||
<tr align=center><td>5</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td bgcolor=yellow>3</td><td bgcolor=yellow> </td><td>-3</td></tr> |
<tr align=center><td>5</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td bgcolor=yellow>3</td><td bgcolor=yellow> </td><td>-3</td></tr> |
||
| Line 41: | Line 39: | ||
<tr align=center><td>-13</td><td bgcolor=yellow> </td><td bgcolor=yellow>3</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td>-3</td></tr> |
<tr align=center><td>-13</td><td bgcolor=yellow> </td><td bgcolor=yellow>3</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td>-3</td></tr> |
||
<tr align=center><td>-15</td><td bgcolor=yellow>1</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td>1</td></tr> |
<tr align=center><td>-15</td><td bgcolor=yellow>1</td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td> </td><td>1</td></tr> |
||
</table> |
</table> | |
||
coloured_jones_2 = <math>q^{10}-4 q^9+2 q^8+14 q^7-23 q^6-8 q^5+54 q^4-41 q^3-47 q^2+106 q-36-103 q^{-1} +143 q^{-2} -12 q^{-3} -148 q^{-4} +148 q^{-5} +19 q^{-6} -158 q^{-7} +117 q^{-8} +38 q^{-9} -123 q^{-10} +66 q^{-11} +33 q^{-12} -65 q^{-13} +27 q^{-14} +14 q^{-15} -22 q^{-16} +9 q^{-17} +3 q^{-18} -4 q^{-19} + q^{-20} </math> | |
|||
{{Computer Talk Header}} |
|||
coloured_jones_3 = <math>q^{21}-4 q^{20}+2 q^{19}+9 q^{18}-26 q^{16}-13 q^{15}+58 q^{14}+43 q^{13}-83 q^{12}-113 q^{11}+95 q^{10}+210 q^9-63 q^8-323 q^7-20 q^6+416 q^5+158 q^4-476 q^3-326 q^2+487 q+494-434 q^{-1} -666 q^{-2} +368 q^{-3} +785 q^{-4} -246 q^{-5} -906 q^{-6} +148 q^{-7} +956 q^{-8} -7 q^{-9} -1001 q^{-10} -100 q^{-11} +966 q^{-12} +231 q^{-13} -908 q^{-14} -310 q^{-15} +769 q^{-16} +375 q^{-17} -610 q^{-18} -380 q^{-19} +433 q^{-20} +332 q^{-21} -268 q^{-22} -259 q^{-23} +155 q^{-24} +163 q^{-25} -75 q^{-26} -94 q^{-27} +47 q^{-28} +37 q^{-29} -24 q^{-30} -19 q^{-31} +23 q^{-32} +3 q^{-33} -12 q^{-34} -2 q^{-35} +5 q^{-36} +3 q^{-37} -4 q^{-38} + q^{-39} </math> | |
|||
coloured_jones_4 = <math>q^{36}-4 q^{35}+2 q^{34}+9 q^{33}-5 q^{32}-3 q^{31}-32 q^{30}+11 q^{29}+70 q^{28}+16 q^{27}-6 q^{26}-192 q^{25}-83 q^{24}+210 q^{23}+235 q^{22}+230 q^{21}-476 q^{20}-576 q^{19}+21 q^{18}+537 q^{17}+1140 q^{16}-235 q^{15}-1220 q^{14}-1023 q^{13}+6 q^{12}+2270 q^{11}+1115 q^{10}-819 q^9-2284 q^8-1952 q^7+2231 q^6+2753 q^5+1209 q^4-2298 q^3-4366 q^2+491 q+3222+3856 q^{-1} -662 q^{-2} -5829 q^{-3} -1973 q^{-4} +2227 q^{-5} +5840 q^{-6} +1676 q^{-7} -6042 q^{-8} -4077 q^{-9} +585 q^{-10} +6878 q^{-11} +3792 q^{-12} -5519 q^{-13} -5562 q^{-14} -1089 q^{-15} +7191 q^{-16} +5500 q^{-17} -4440 q^{-18} -6449 q^{-19} -2802 q^{-20} +6586 q^{-21} +6689 q^{-22} -2562 q^{-23} -6251 q^{-24} -4374 q^{-25} +4635 q^{-26} +6680 q^{-27} -196 q^{-28} -4476 q^{-29} -4890 q^{-30} +1881 q^{-31} +4953 q^{-32} +1370 q^{-33} -1845 q^{-34} -3733 q^{-35} -122 q^{-36} +2403 q^{-37} +1347 q^{-38} -2 q^{-39} -1820 q^{-40} -576 q^{-41} +631 q^{-42} +533 q^{-43} +437 q^{-44} -530 q^{-45} -252 q^{-46} +51 q^{-47} +22 q^{-48} +230 q^{-49} -95 q^{-50} -17 q^{-51} -62 q^{-53} +59 q^{-54} -19 q^{-55} +17 q^{-56} +9 q^{-57} -23 q^{-58} +8 q^{-59} -6 q^{-60} +5 q^{-61} +3 q^{-62} -4 q^{-63} + q^{-64} </math> | |
|||
<table> |
|||
coloured_jones_5 = <math>q^{55}-4 q^{54}+2 q^{53}+9 q^{52}-5 q^{51}-8 q^{50}-9 q^{49}-8 q^{48}+22 q^{47}+63 q^{46}+22 q^{45}-74 q^{44}-133 q^{43}-115 q^{42}+65 q^{41}+317 q^{40}+394 q^{39}+44 q^{38}-524 q^{37}-848 q^{36}-545 q^{35}+472 q^{34}+1534 q^{33}+1564 q^{32}+104 q^{31}-1937 q^{30}-2976 q^{29}-1751 q^{28}+1492 q^{27}+4402 q^{26}+4276 q^{25}+445 q^{24}-4706 q^{23}-7184 q^{22}-4190 q^{21}+3047 q^{20}+9219 q^{19}+9027 q^{18}+1320 q^{17}-8945 q^{16}-13720 q^{15}-8006 q^{14}+5306 q^{13}+16469 q^{12}+15821 q^{11}+1851 q^{10}-15820 q^9-22871 q^8-11687 q^7+11014 q^6+27476 q^5+22575 q^4-2495 q^3-28442 q^2-32575 q-8697+25475 q^{-1} +40565 q^{-2} +20767 q^{-3} -19455 q^{-4} -45362 q^{-5} -32362 q^{-6} +11130 q^{-7} +47609 q^{-8} +42408 q^{-9} -2411 q^{-10} -47264 q^{-11} -50446 q^{-12} -6411 q^{-13} +45857 q^{-14} +56709 q^{-15} +13975 q^{-16} -43492 q^{-17} -61496 q^{-18} -20995 q^{-19} +41233 q^{-20} +65425 q^{-21} +27032 q^{-22} -38415 q^{-23} -68688 q^{-24} -33287 q^{-25} +35262 q^{-26} +71176 q^{-27} +39436 q^{-28} -30398 q^{-29} -72336 q^{-30} -46097 q^{-31} +23873 q^{-32} +71274 q^{-33} +52009 q^{-34} -14921 q^{-35} -66993 q^{-36} -56686 q^{-37} +4579 q^{-38} +59125 q^{-39} +58319 q^{-40} +6144 q^{-41} -47851 q^{-42} -56204 q^{-43} -15438 q^{-44} +34582 q^{-45} +50059 q^{-46} +21632 q^{-47} -21187 q^{-48} -40637 q^{-49} -23983 q^{-50} +9608 q^{-51} +29845 q^{-52} +22500 q^{-53} -1315 q^{-54} -19347 q^{-55} -18472 q^{-56} -3367 q^{-57} +10885 q^{-58} +13263 q^{-59} +4988 q^{-60} -4934 q^{-61} -8507 q^{-62} -4597 q^{-63} +1606 q^{-64} +4668 q^{-65} +3401 q^{-66} +46 q^{-67} -2334 q^{-68} -2126 q^{-69} -429 q^{-70} +904 q^{-71} +1132 q^{-72} +495 q^{-73} -301 q^{-74} -557 q^{-75} -296 q^{-76} +49 q^{-77} +204 q^{-78} +169 q^{-79} +35 q^{-80} -72 q^{-81} -88 q^{-82} -15 q^{-83} +17 q^{-84} +12 q^{-85} +27 q^{-86} +3 q^{-87} -17 q^{-88} -3 q^{-89} +4 q^{-90} -6 q^{-91} +5 q^{-92} +3 q^{-93} -4 q^{-94} + q^{-95} </math> | |
|||
<tr valign=top> |
|||
coloured_jones_6 = <math>q^{78}-4 q^{77}+2 q^{76}+9 q^{75}-5 q^{74}-8 q^{73}-14 q^{72}+15 q^{71}+3 q^{70}+15 q^{69}+68 q^{68}-26 q^{67}-86 q^{66}-153 q^{65}-21 q^{64}+56 q^{63}+210 q^{62}+491 q^{61}+190 q^{60}-250 q^{59}-903 q^{58}-836 q^{57}-620 q^{56}+367 q^{55}+2093 q^{54}+2348 q^{53}+1470 q^{52}-1324 q^{51}-3293 q^{50}-5067 q^{49}-3686 q^{48}+1697 q^{47}+6581 q^{46}+9489 q^{45}+6049 q^{44}-35 q^{43}-10547 q^{42}-16538 q^{41}-12353 q^{40}-932 q^{39}+14894 q^{38}+23370 q^{37}+23945 q^{36}+5342 q^{35}-18963 q^{34}-35541 q^{33}-35784 q^{32}-13872 q^{31}+17450 q^{30}+51592 q^{29}+54149 q^{28}+28028 q^{27}-18441 q^{26}-63016 q^{25}-78088 q^{24}-53589 q^{23}+17336 q^{22}+79351 q^{21}+108954 q^{20}+77740 q^{19}-4510 q^{18}-98846 q^{17}-152582 q^{16}-107807 q^{15}-3599 q^{14}+124933 q^{13}+192532 q^{12}+152823 q^{11}+7525 q^{10}-166458 q^9-241469 q^8-188271 q^7+217 q^6+206600 q^5+308987 q^4+214477 q^3-33219 q^2-265788 q-363557-220510 q^{-1} +75072 q^{-2} +353982 q^{-3} +406834 q^{-4} +188234 q^{-5} -153273 q^{-6} -431978 q^{-7} -423976 q^{-8} -133940 q^{-9} +275403 q^{-10} +501456 q^{-11} +391334 q^{-12} +24539 q^{-13} -392844 q^{-14} -541057 q^{-15} -323845 q^{-16} +142871 q^{-17} +505015 q^{-18} +519968 q^{-19} +183718 q^{-20} -310007 q^{-21} -582336 q^{-22} -450631 q^{-23} +25931 q^{-24} +473028 q^{-25} +587365 q^{-26} +292811 q^{-27} -238220 q^{-28} -595039 q^{-29} -531071 q^{-30} -57346 q^{-31} +445995 q^{-32} +634805 q^{-33} +374947 q^{-34} -181645 q^{-35} -607338 q^{-36} -604029 q^{-37} -139053 q^{-38} +414046 q^{-39} +680395 q^{-40} +469528 q^{-41} -97920 q^{-42} -595466 q^{-43} -678658 q^{-44} -259600 q^{-45} +324454 q^{-46} +686103 q^{-47} +575432 q^{-48} +52303 q^{-49} -497136 q^{-50} -701406 q^{-51} -403747 q^{-52} +142621 q^{-53} +581643 q^{-54} +621571 q^{-55} +235112 q^{-56} -287062 q^{-57} -596395 q^{-58} -481338 q^{-59} -75312 q^{-60} +355455 q^{-61} +529521 q^{-62} +342567 q^{-63} -44939 q^{-64} -369190 q^{-65} -415401 q^{-66} -208035 q^{-67} +108338 q^{-68} +322841 q^{-69} +304969 q^{-70} +102154 q^{-71} -135601 q^{-72} -246291 q^{-73} -198805 q^{-74} -36886 q^{-75} +122951 q^{-76} +177133 q^{-77} +115245 q^{-78} -4372 q^{-79} -91715 q^{-80} -112645 q^{-81} -61507 q^{-82} +17368 q^{-83} +65883 q^{-84} +64252 q^{-85} +24702 q^{-86} -15829 q^{-87} -40520 q^{-88} -34699 q^{-89} -8179 q^{-90} +14459 q^{-91} +22294 q^{-92} +14378 q^{-93} +2588 q^{-94} -9363 q^{-95} -12103 q^{-96} -5644 q^{-97} +1258 q^{-98} +5297 q^{-99} +4536 q^{-100} +2604 q^{-101} -1348 q^{-102} -3173 q^{-103} -1815 q^{-104} -316 q^{-105} +938 q^{-106} +949 q^{-107} +1020 q^{-108} -44 q^{-109} -699 q^{-110} -399 q^{-111} -182 q^{-112} +113 q^{-113} +98 q^{-114} +292 q^{-115} +43 q^{-116} -125 q^{-117} -49 q^{-118} -46 q^{-119} +12 q^{-120} -20 q^{-121} +58 q^{-122} +13 q^{-123} -23 q^{-124} +3 q^{-125} -7 q^{-126} +4 q^{-127} -6 q^{-128} +5 q^{-129} +3 q^{-130} -4 q^{-131} + q^{-132} </math> | |
|||
<td><pre style="color: blue; border: 0px; padding: 0em">In[1]:= </pre></td> |
|||
coloured_jones_7 = <math>q^{105}-4 q^{104}+2 q^{103}+9 q^{102}-5 q^{101}-8 q^{100}-14 q^{99}+10 q^{98}+26 q^{97}-4 q^{96}+20 q^{95}+20 q^{94}-39 q^{93}-86 q^{92}-130 q^{91}-18 q^{90}+189 q^{89}+218 q^{88}+307 q^{87}+213 q^{86}-170 q^{85}-575 q^{84}-1114 q^{83}-937 q^{82}+19 q^{81}+1068 q^{80}+2323 q^{79}+2755 q^{78}+1680 q^{77}-476 q^{76}-4106 q^{75}-6650 q^{74}-6050 q^{73}-2726 q^{72}+4089 q^{71}+10866 q^{70}+14273 q^{69}+12544 q^{68}+2272 q^{67}-12339 q^{66}-24453 q^{65}-29328 q^{64}-19717 q^{63}+1745 q^{62}+27496 q^{61}+49490 q^{60}+51247 q^{59}+29082 q^{58}-11109 q^{57}-58147 q^{56}-86351 q^{55}-81674 q^{54}-40221 q^{53}+32573 q^{52}+103269 q^{51}+141541 q^{50}+125723 q^{49}+45576 q^{48}-66572 q^{47}-170667 q^{46}-221936 q^{45}-178291 q^{44}-51085 q^{43}+122230 q^{42}+276101 q^{41}+328024 q^{40}+248280 q^{39}+44859 q^{38}-219684 q^{37}-424559 q^{36}-479667 q^{35}-329249 q^{34}+1427 q^{33}+377537 q^{32}+650984 q^{31}+674467 q^{30}+385742 q^{29}-116839 q^{28}-651507 q^{27}-969702 q^{26}-876873 q^{25}-371829 q^{24}+388842 q^{23}+1079864 q^{22}+1348069 q^{21}+1026443 q^{20}+165462 q^{19}-892107 q^{18}-1646414 q^{17}-1716158 q^{16}-958013 q^{15}+358674 q^{14}+1638995 q^{13}+2276578 q^{12}+1862041 q^{11}+484452 q^{10}-1256298 q^9-2561391 q^8-2712791 q^7-1522784 q^6+513794 q^5+2479981 q^4+3353864 q^3+2601296 q^2+498263 q-2022845-3681823 q^{-1} -3565159 q^{-2} -1639523 q^{-3} +1255163 q^{-4} +3659966 q^{-5} +4297269 q^{-6} +2766712 q^{-7} -288310 q^{-8} -3324924 q^{-9} -4744622 q^{-10} -3758569 q^{-11} -745625 q^{-12} +2758223 q^{-13} +4907479 q^{-14} +4544110 q^{-15} +1737138 q^{-16} -2065024 q^{-17} -4840920 q^{-18} -5101061 q^{-19} -2600896 q^{-20} +1345575 q^{-21} +4615074 q^{-22} +5448137 q^{-23} +3301323 q^{-24} -677090 q^{-25} -4314371 q^{-26} -5632970 q^{-27} -3829595 q^{-28} +110075 q^{-29} +4003226 q^{-30} +5712096 q^{-31} +4213556 q^{-32} +338862 q^{-33} -3735338 q^{-34} -5745173 q^{-35} -4491455 q^{-36} -677796 q^{-37} +3532924 q^{-38} +5780653 q^{-39} +4718685 q^{-40} +940126 q^{-41} -3399370 q^{-42} -5851981 q^{-43} -4945050 q^{-44} -1177198 q^{-45} +3304370 q^{-46} +5969456 q^{-47} +5218300 q^{-48} +1449833 q^{-49} -3198548 q^{-50} -6111765 q^{-51} -5558109 q^{-52} -1818171 q^{-53} +3006066 q^{-54} +6225844 q^{-55} +5956977 q^{-56} +2321108 q^{-57} -2654449 q^{-58} -6228347 q^{-59} -6356639 q^{-60} -2958913 q^{-61} +2081549 q^{-62} +6023339 q^{-63} +6666957 q^{-64} +3679256 q^{-65} -1277096 q^{-66} -5532402 q^{-67} -6768298 q^{-68} -4374923 q^{-69} +287316 q^{-70} +4722091 q^{-71} +6558152 q^{-72} +4910362 q^{-73} +771904 q^{-74} -3634733 q^{-75} -5980942 q^{-76} -5151849 q^{-77} -1743975 q^{-78} +2384254 q^{-79} +5059882 q^{-80} +5018303 q^{-81} +2473724 q^{-82} -1138645 q^{-83} -3903117 q^{-84} -4509092 q^{-85} -2849932 q^{-86} +70836 q^{-87} +2672182 q^{-88} +3709833 q^{-89} +2847577 q^{-90} +692290 q^{-91} -1543588 q^{-92} -2768946 q^{-93} -2524928 q^{-94} -1100025 q^{-95} +650464 q^{-96} +1846579 q^{-97} +2004985 q^{-98} +1188097 q^{-99} -55431 q^{-100} -1072392 q^{-101} -1427253 q^{-102} -1050542 q^{-103} -255713 q^{-104} +512977 q^{-105} +906860 q^{-106} +801291 q^{-107} +350733 q^{-108} -169154 q^{-109} -508679 q^{-110} -538782 q^{-111} -318086 q^{-112} -1268 q^{-113} +247773 q^{-114} +321322 q^{-115} +232766 q^{-116} +60507 q^{-117} -99750 q^{-118} -171068 q^{-119} -147523 q^{-120} -62536 q^{-121} +30928 q^{-122} +81618 q^{-123} +81789 q^{-124} +44758 q^{-125} -4126 q^{-126} -35039 q^{-127} -41684 q^{-128} -26819 q^{-129} -2055 q^{-130} +14365 q^{-131} +19478 q^{-132} +13821 q^{-133} +2242 q^{-134} -5527 q^{-135} -8897 q^{-136} -6990 q^{-137} -1259 q^{-138} +2497 q^{-139} +4247 q^{-140} +3300 q^{-141} +370 q^{-142} -1068 q^{-143} -1866 q^{-144} -1680 q^{-145} -298 q^{-146} +445 q^{-147} +1047 q^{-148} +895 q^{-149} +11 q^{-150} -214 q^{-151} -358 q^{-152} -390 q^{-153} -94 q^{-154} + q^{-155} +207 q^{-156} +237 q^{-157} -17 q^{-158} -23 q^{-159} -45 q^{-160} -59 q^{-161} -9 q^{-162} -25 q^{-163} +26 q^{-164} +44 q^{-165} -13 q^{-166} -3 q^{-167} - q^{-168} -7 q^{-169} +4 q^{-170} -6 q^{-171} +5 q^{-172} +3 q^{-173} -4 q^{-174} + q^{-175} </math> | |
|||
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
|||
computer_talk = |
|||
</tr> |
|||
<table> |
|||
<tr valign=top><td colspan=2><pre style="border: 0px; padding: 0em">Loading KnotTheory` (version of August 17, 2005, 14:44:34)...</pre></td></tr> |
|||
<tr valign=top> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[2]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Crossings[Knot[10, 112]]</nowiki></pre></td></tr> |
|||
<td><pre style="color: blue; border: 0px; padding: 0em">In[1]:= </pre></td> |
|||
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
|||
</tr> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[3]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>PD[X[6, 2, 7, 1], X[8, 3, 9, 4], X[18, 11, 19, 12], X[20, 13, 1, 14], |
|||
<tr valign=top><td colspan=2><nowiki>Loading KnotTheory` (version of August 29, 2005, 15:33:11)...</nowiki></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[2]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>PD[Knot[10, 112]]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[2]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>PD[X[6, 2, 7, 1], X[8, 3, 9, 4], X[18, 11, 19, 12], X[20, 13, 1, 14], |
|||
X[2, 16, 3, 15], X[4, 17, 5, 18], X[12, 19, 13, 20], X[10, 6, 11, 5], |
X[2, 16, 3, 15], X[4, 17, 5, 18], X[12, 19, 13, 20], X[10, 6, 11, 5], |
||
X[14, 7, 15, 8], X[16, 10, 17, 9]]</nowiki></ |
X[14, 7, 15, 8], X[16, 10, 17, 9]]</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[4]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>GaussCode[Knot[10, 112]]</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[4]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>GaussCode[1, -5, 2, -6, 8, -1, 9, -2, 10, -8, 3, -7, 4, -9, 5, -10, 6, |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[3]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>GaussCode[Knot[10, 112]]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[3]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>GaussCode[1, -5, 2, -6, 8, -1, 9, -2, 10, -8, 3, -7, 4, -9, 5, -10, 6, |
|||
-3, 7, -4]</nowiki></ |
-3, 7, -4]</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[5]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>BR[Knot[10, 112]]</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[5]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>BR[3, {-1, -1, -1, 2, -1, 2, -1, 2, -1, 2}]</nowiki></pre></td></tr> |
|||
< |
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[4]:=</code></td> |
||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>DTCode[Knot[10, 112]]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[4]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>DTCode[6, 8, 10, 14, 16, 18, 20, 2, 4, 12]</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[5]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>br = BR[Knot[10, 112]]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[5]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>BR[3, {-1, -1, -1, 2, -1, 2, -1, 2, -1, 2}]</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[6]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{First[br], Crossings[br]}</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[6]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{3, 10}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[7]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>BraidIndex[Knot[10, 112]]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[7]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>3</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[8]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Show[DrawMorseLink[Knot[10, 112]]]</nowiki></code></td></tr> |
|||
<tr align=left><td></td><td>[[Image:10_112_ML.gif]]</td></tr><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[8]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>-Graphics-</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[9]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> (#[Knot[10, 112]]&) /@ { |
|||
SymmetryType, UnknottingNumber, ThreeGenus, |
|||
BridgeIndex, SuperBridgeIndex, NakanishiIndex |
|||
}</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[9]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{Reversible, 2, 4, 3, NotAvailable, 1}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[10]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>alex = Alexander[Knot[10, 112]][t]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[10]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> -4 5 11 17 2 3 4 |
|||
-19 - t + -- - -- + -- + 17 t - 11 t + 5 t - t |
-19 - t + -- - -- + -- + 17 t - 11 t + 5 t - t |
||
3 2 t |
3 2 t |
||
t t</nowiki></ |
t t</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[7]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Conway[Knot[10, 112]][z]</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[7]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki> 2 4 6 8 |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[11]:=</code></td> |
|||
1 + 2 z - z - 3 z - z</nowiki></pre></td></tr> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Conway[Knot[10, 112]][z]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[8]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>{Knot[10, 112], Knot[11, Alternating, 184]}</nowiki></pre></td></tr> |
|||
< |
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[11]:=</code></td> |
||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> 2 4 6 8 |
|||
1 + 2 z - z - 3 z - z</nowiki></code></td></tr> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[10]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>J=Jones[Knot[10, 112]][q]</nowiki></pre></td></tr> |
|||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[10]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki> -7 4 7 11 14 14 14 2 3 |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[12]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Select[AllKnots[], (alex === Alexander[#][t])&]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[12]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{Knot[10, 112], Knot[11, Alternating, 184]}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[13]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{KnotDet[Knot[10, 112]], KnotSignature[Knot[10, 112]]}</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[13]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{87, -2}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[14]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Jones[Knot[10, 112]][q]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[14]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> -7 4 7 11 14 14 14 2 3 |
|||
-10 + q - -- + -- - -- + -- - -- + -- + 7 q - 4 q + q |
-10 + q - -- + -- - -- + -- - -- + -- + 7 q - 4 q + q |
||
6 5 4 3 2 q |
6 5 4 3 2 q |
||
q q q q q</nowiki></ |
q q q q q</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[11]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Select[AllKnots[], (J === Jones[#][q] || (J /. q-> 1/q) === Jones[#][q])&]</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[11]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>{Knot[10, 112]}</nowiki></pre></td></tr> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[15]:=</code></td> |
|||
<math>\textrm{Include}(\textrm{ColouredJonesM.mhtml})</math> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Select[AllKnots[], (J === Jones[#][q] || (J /. q-> 1/q) === Jones[#][q])&]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[12]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki> -20 2 -16 3 -12 2 -8 6 -4 3 2 |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[15]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{Knot[10, 112]}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[16]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>A2Invariant[Knot[10, 112]][q]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[16]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> -20 2 -16 3 -12 2 -8 6 -4 3 2 |
|||
q - --- + q - --- - q + --- - q + -- - q + -- - 2 q + |
q - --- + q - --- - q + --- - q + -- - q + -- - 2 q + |
||
18 14 10 6 2 |
18 14 10 6 2 |
||
| Line 91: | Line 179: | ||
4 6 8 |
4 6 8 |
||
q - 2 q + q</nowiki></ |
q - 2 q + q</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[13]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Kauffman[Knot[10, 112]][a, z]</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[13]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki> 2 4 3 5 2 2 2 4 2 6 2 |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[17]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>HOMFLYPT[Knot[10, 112]][a, z]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[17]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> 2 4 2 4 2 4 2 4 4 4 6 |
|||
-1 + 4 a - 2 a + z + a z + 3 z - 7 a z + 3 a z + z - |
|||
2 6 4 6 2 8 |
|||
5 a z + a z - a z</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[18]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Kauffman[Knot[10, 112]][a, z]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[18]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> 2 4 3 5 2 2 2 4 2 6 2 |
|||
-1 - 4 a - 2 a + 2 a z + 2 a z - 3 z - 3 a z + a z + a z + |
-1 - 4 a - 2 a + 2 a z + 2 a z - 3 z - 3 a z + a z + a z + |
||
| Line 119: | Line 223: | ||
3 9 |
3 9 |
||
3 a z</nowiki></ |
3 a z</nowiki></code></td></tr> |
||
</table> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[14]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>{Vassiliev[2][Knot[10, 112]], Vassiliev[3][Knot[10, 112]]}</nowiki></pre></td></tr> |
|||
<table><tr align=left> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[14]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>{0, -2}</nowiki></pre></td></tr> |
|||
< |
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[19]:=</code></td> |
||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{Vassiliev[2][Knot[10, 112]], Vassiliev[3][Knot[10, 112]]}</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[19]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>{2, -2}</nowiki></code></td></tr> |
|||
</table> |
|||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[20]:=</code></td> |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>Kh[Knot[10, 112]][q, t]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[20]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>7 8 1 3 1 4 3 7 4 |
|||
-- + - + ------ + ------ + ------ + ------ + ----- + ----- + ----- + |
-- + - + ------ + ------ + ------ + ------ + ----- + ----- + ----- + |
||
3 q 15 6 13 5 11 5 11 4 9 4 9 3 7 3 |
3 q 15 6 13 5 11 5 11 4 9 4 9 3 7 3 |
||
| Line 134: | Line 248: | ||
3 3 5 3 7 4 |
3 3 5 3 7 4 |
||
q t + 3 q t + q t</nowiki></ |
q t + 3 q t + q t</nowiki></code></td></tr> |
||
</table> |
</table> |
||
<table><tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">In[21]:=</code></td> |
|||
[[Category:Knot Page]] |
|||
<td><code style="white-space: pre; color: red; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki>ColouredJones[Knot[10, 112], 2][q]</nowiki></code></td></tr> |
|||
<tr align=left> |
|||
<td width=70px><code style="color: blue; border: 0px; padding: 0em">Out[21]:=</code></td> |
|||
<td><code style="white-space: pre; color: black; border: 0px; padding: 0em; background-color: rgb(255,255,255);"><nowiki> -20 4 3 9 22 14 27 65 33 66 |
|||
-36 + q - --- + --- + --- - --- + --- + --- - --- + --- + --- - |
|||
19 18 17 16 15 14 13 12 11 |
|||
q q q q q q q q q |
|||
123 38 117 158 19 148 148 12 143 103 |
|||
--- + -- + --- - --- + -- + --- - --- - -- + --- - --- + 106 q - |
|||
10 9 8 7 6 5 4 3 2 q |
|||
q q q q q q q q q |
|||
2 3 4 5 6 7 8 9 10 |
|||
47 q - 41 q + 54 q - 8 q - 23 q + 14 q + 2 q - 4 q + q</nowiki></code></td></tr> |
|||
</table> }} |
|||
Latest revision as of 17:05, 1 September 2005
|
|
|
 (KnotPlot image) |
See the full Rolfsen Knot Table. Visit 10 112's page at the Knot Server (KnotPlot driven, includes 3D interactive images!) |
Knot presentations
| Planar diagram presentation | X6271 X8394 X18,11,19,12 X20,13,1,14 X2,16,3,15 X4,17,5,18 X12,19,13,20 X10,6,11,5 X14,7,15,8 X16,10,17,9 |
| Gauss code | 1, -5, 2, -6, 8, -1, 9, -2, 10, -8, 3, -7, 4, -9, 5, -10, 6, -3, 7, -4 |
| Dowker-Thistlethwaite code | 6 8 10 14 16 18 20 2 4 12 |
| Conway Notation | [8*3] |
| Minimum Braid Representative | A Morse Link Presentation | An Arc Presentation | |||
Length is 10, width is 3, Braid index is 3 |
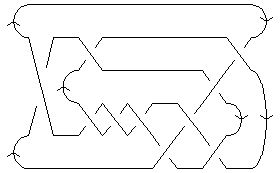
|
 [{3, 12}, {2, 10}, {4, 11}, {5, 3}, {6, 4}, {7, 5}, {1, 6}, {9, 2}, {10, 8}, {12, 9}, {11, 7}, {8, 1}] |
[edit Notes on presentations of 10 112]
KnotTheory`. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of May 31, 2006, 14:15:20.091.
|
In[3]:=
|
K = Knot["10 112"];
|
In[4]:=
|
PD[K]
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
Out[4]=
|
X6271 X8394 X18,11,19,12 X20,13,1,14 X2,16,3,15 X4,17,5,18 X12,19,13,20 X10,6,11,5 X14,7,15,8 X16,10,17,9 |
In[5]:=
|
GaussCode[K]
|
Out[5]=
|
1, -5, 2, -6, 8, -1, 9, -2, 10, -8, 3, -7, 4, -9, 5, -10, 6, -3, 7, -4 |
In[6]:=
|
DTCode[K]
|
Out[6]=
|
6 8 10 14 16 18 20 2 4 12 |
(The path below may be different on your system)
In[7]:=
|
AppendTo[$Path, "C:/bin/LinKnot/"];
|
In[8]:=
|
ConwayNotation[K]
|
Out[8]=
|
[8*3] |
In[9]:=
|
br = BR[K]
|
KnotTheory::credits: The minimum braids representing the knots with up to 10 crossings were provided by Thomas Gittings. See arXiv:math.GT/0401051.
|
Out[9]=
|
In[10]:=
|
{First[br], Crossings[br], BraidIndex[K]}
|
KnotTheory::credits: The braid index data known to KnotTheory` is taken from Charles Livingston's http://www.indiana.edu/~knotinfo/.
|
KnotTheory::loading: Loading precomputed data in IndianaData`.
|
Out[10]=
|
{ 3, 10, 3 } |
In[11]:=
|
Show[BraidPlot[br]]
|
Out[11]=
|
-Graphics- |
In[12]:=
|
Show[DrawMorseLink[K]]
|
KnotTheory::credits: "MorseLink was added to KnotTheory` by Siddarth Sankaran at the University of Toronto in the summer of 2005."
|
KnotTheory::credits: "DrawMorseLink was written by Siddarth Sankaran at the University of Toronto in the summer of 2005."
|

|
Out[12]=
|
-Graphics- |
In[13]:=
|
ap = ArcPresentation[K]
|
Out[13]=
|
ArcPresentation[{3, 12}, {2, 10}, {4, 11}, {5, 3}, {6, 4}, {7, 5}, {1, 6}, {9, 2}, {10, 8}, {12, 9}, {11, 7}, {8, 1}] |
In[14]:=
|
Draw[ap]
|

|
Out[14]=
|
-Graphics- |
Three dimensional invariants
|
Four dimensional invariants
|
Polynomial invariants
A1 Invariants.
| Weight | Invariant |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 |
A2 Invariants.
| Weight | Invariant |
|---|---|
| 1,0 | |
| 1,1 | |
| 2,0 |
A3 Invariants.
| Weight | Invariant |
|---|---|
| 0,1,0 | |
| 1,0,0 | |
| 1,0,1 |
A4 Invariants.
| Weight | Invariant |
|---|---|
| 0,1,0,0 | |
| 1,0,0,0 |
B2 Invariants.
| Weight | Invariant |
|---|---|
| 0,1 | |
| 1,0 |
D4 Invariants.
| Weight | Invariant |
|---|---|
| 1,0,0,0 |
G2 Invariants.
| Weight | Invariant |
|---|---|
| 1,0 |
.
KnotTheory`, as shown in the (simulated) Mathematica session below. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting. This Mathematica session is also available (albeit only for the knot 5_2) as the notebook PolynomialInvariantsSession.nb.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of August 31, 2006, 11:25:27.5625.
|
In[3]:=
|
K = Knot["10 112"];
|
In[4]:=
|
Alexander[K][t]
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
Out[4]=
|
In[5]:=
|
Conway[K][z]
|
Out[5]=
|
In[6]:=
|
Alexander[K, 2][t]
|
KnotTheory::credits: The program Alexander[K, r] to compute Alexander ideals was written by Jana Archibald at the University of Toronto in the summer of 2005.
|
Out[6]=
|
In[7]:=
|
{KnotDet[K], KnotSignature[K]}
|
Out[7]=
|
{ 87, -2 } |
In[8]:=
|
Jones[K][q]
|
KnotTheory::loading: Loading precomputed data in Jones4Knots`.
|
Out[8]=
|
In[9]:=
|
HOMFLYPT[K][a, z]
|
KnotTheory::credits: The HOMFLYPT program was written by Scott Morrison.
|
Out[9]=
|
In[10]:=
|
Kauffman[K][a, z]
|
KnotTheory::loading: Loading precomputed data in Kauffman4Knots`.
|
Out[10]=
|
"Similar" Knots (within the Atlas)
Same Alexander/Conway Polynomial: {K11a184,}
Same Jones Polynomial (up to mirroring, ): {}
KnotTheory`. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of May 31, 2006, 14:15:20.091.
|
In[3]:=
|
K = Knot["10 112"];
|
In[4]:=
|
{A = Alexander[K][t], J = Jones[K][q]}
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
KnotTheory::loading: Loading precomputed data in Jones4Knots`.
|
Out[4]=
|
{ , } |
In[5]:=
|
DeleteCases[Select[AllKnots[], (A === Alexander[#][t]) &], K]
|
KnotTheory::loading: Loading precomputed data in DTCode4KnotsTo11`.
|
KnotTheory::credits: The GaussCode to PD conversion was written by Siddarth Sankaran at the University of Toronto in the summer of 2005.
|
Out[5]=
|
{K11a184,} |
In[6]:=
|
DeleteCases[
Select[
AllKnots[],
(J === Jones[#][q] || (J /. q -> 1/q) === Jones[#][q]) &
],
K
]
|
KnotTheory::loading: Loading precomputed data in Jones4Knots11`.
|
Out[6]=
|
{} |
Vassiliev invariants
| V2 and V3: | (2, -2) |
| V2,1 through V6,9: |
|
V2,1 through V6,9 were provided by Petr Dunin-Barkowski <barkovs@itep.ru>, Andrey Smirnov <asmirnov@itep.ru>, and Alexei Sleptsov <sleptsov@itep.ru> and uploaded on October 2010 by User:Drorbn. Note that they are normalized differently than V2 and V3.
Khovanov Homology
| The coefficients of the monomials are shown, along with their alternating sums (fixed , alternation over ). The squares with yellow highlighting are those on the "critical diagonals", where or , where -2 is the signature of 10 112. Nonzero entries off the critical diagonals (if any exist) are highlighted in red. |
|
| Integral Khovanov Homology
(db, data source) |
|
The Coloured Jones Polynomials
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 |
Computer Talk
Much of the above data can be recomputed by Mathematica using the package KnotTheory`. See A Sample KnotTheory` Session, or any of the Computer Talk sections above.
Modifying This Page
| Read me first: Modifying Knot Pages
See/edit the Rolfsen Knot Page master template (intermediate). See/edit the Rolfsen_Splice_Base (expert). Back to the top. |
|