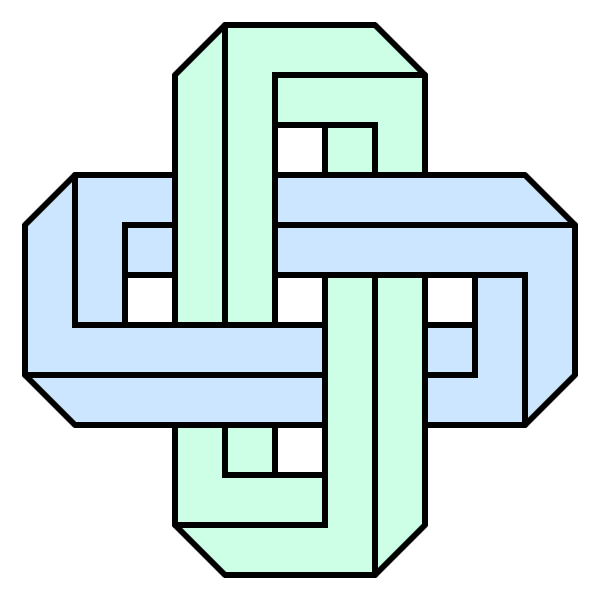L4a1

L2a1

L5a1

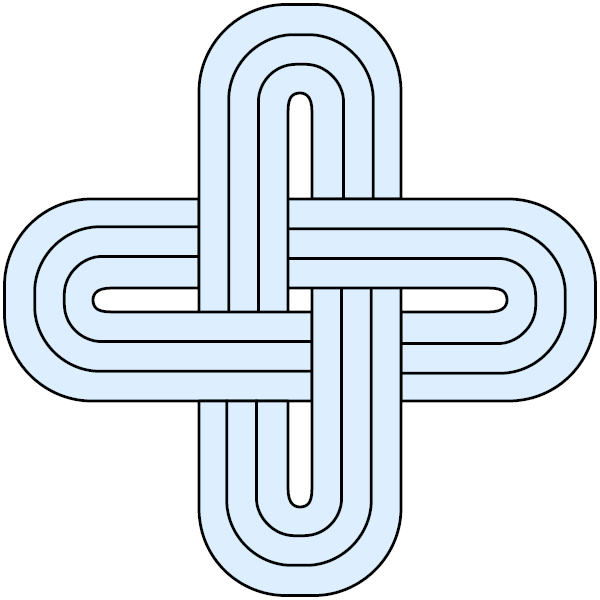
(Knotscape image)	See the full Thistlethwaite Link Table (up to 11 crossings). Visit L4a1 at Knotilus!
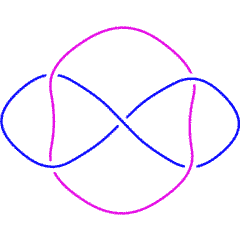
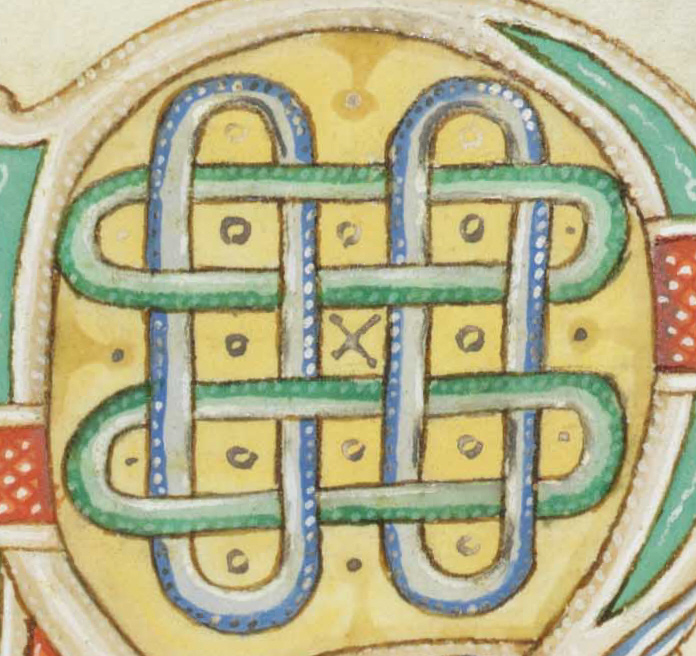
	L4a1 is $4_{1}^{2}$ in the Rolfsen table of links. It frequently occurs in late Roman mosaics and some medieval decorations. In this context, it is called the "Solomon's knot" (sigillum Salomonis) or "guilloche knot". It is also the "Kramo-bone" symbol (meaning "one being bad makes all appear to be bad") of the Adinkra symbol system. Link L10a101 contains multiple L4a1 configurations.

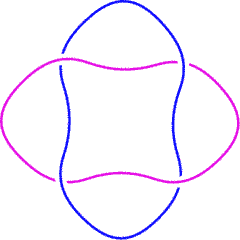
Simple squared depiction		A Kolam with two cycles[1]		Hearst Castle tile [2]		Mosaic seen at Kibbutz Lahav [3]
Carving above door of church in Italy		Decorative depiction		(crossings along one side)		Linked hearts used as symbol of Vendée region of France
Heraldic ornament.		Composed of intersecting circles.		Decorative fitting closely within square.		Ancient Roman mosaic.
Made of two (impossible) Penrose rectangles.		Array of "Solomon's knots" forming overall circular patterns.		Configuration of three L4a1		Configuration of four L4a1
Medieval manuscript		Medieval manuscript		Medieval manuscript		Rotated knotwork cross with eight L4a1 sub-configurations
Knotopologynn-diagram for "Solomon's knots"		Commercial logo

Link Presentations

[edit Notes on L4a1's Link Presentations] Why such an ugly Braid Representative?

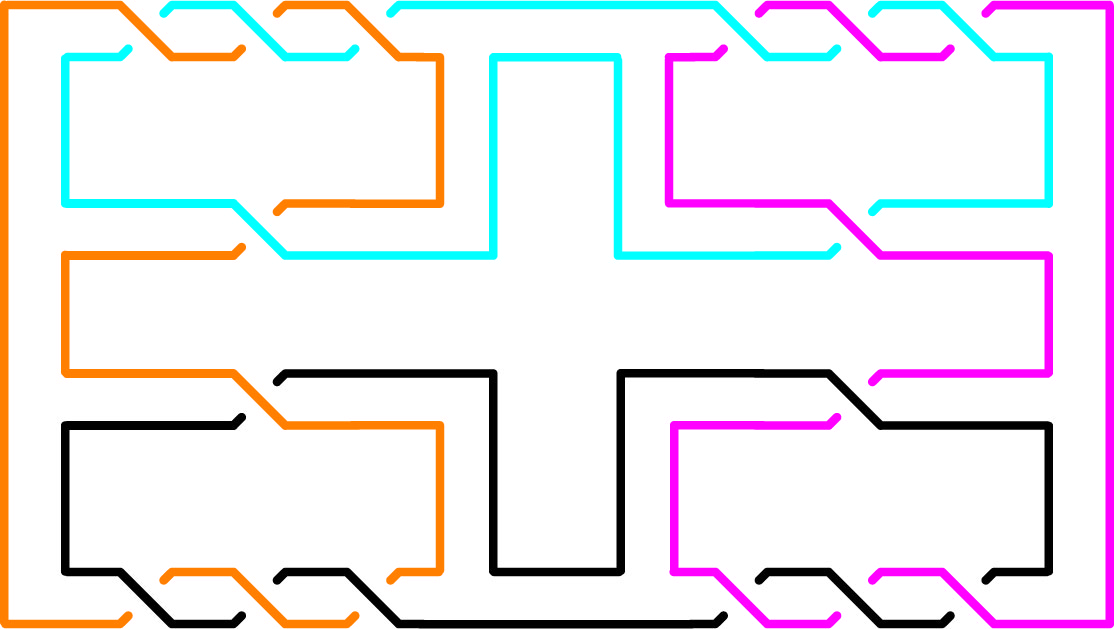
Planar diagram presentation	X6172 X8354 X2536 X4718
Gauss code	{1, -3, 2, -4}, {3, -1, 4, -2}

A Braid Representative
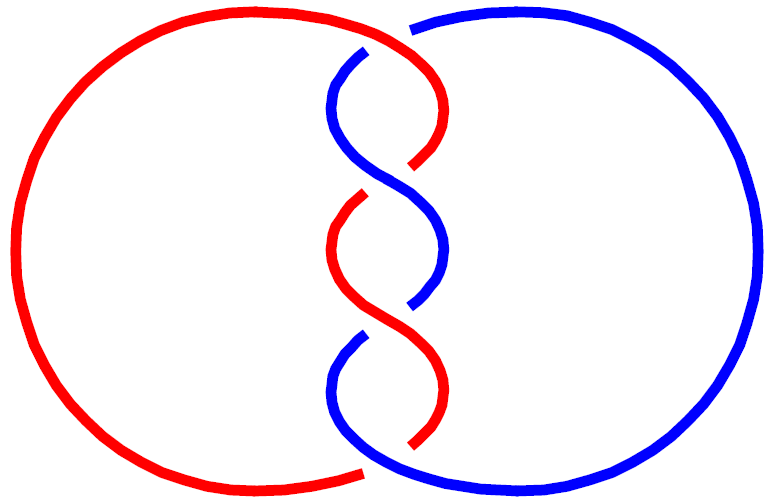
A Morse Link Presentation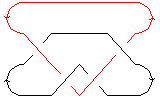

Polynomial invariants

Multivariable Alexander Polynomial (in $u$, $v$, $w$, ...)	${\frac {-u-v}{{\sqrt {u}}{\sqrt {v}}}}$ (db)
Jones polynomial	$-{\frac {1}{q^{9/2}}}-{\frac {1}{q^{5/2}}}+{\frac {1}{q^{3/2}}}-{\frac {1}{\sqrt {q}}}$ (db)
Signature	-1 (db)
HOMFLY-PT polynomial	$a^{5}z^{-1}-a^{3}z-a^{3}z^{-1}-az$ (db)
Kauffman polynomial	$a^{5}z^{3}-3a^{5}z+a^{5}z^{-1}+a^{4}z^{2}-a^{4}+a^{3}z^{3}-2a^{3}z+a^{3}z^{-1}+a^{2}z^{2}+az$ (db)

Khovanov Homology

The coefficients of the monomials $t^{r}q^{j}$ are shown, along with their alternating sums $\chi$ (fixed $j$, alternation over $r$).

\   r    \    j   \ -4 -3 -2 -1 0 χ 0         1 1 -2       1 1 0 -4           0 -6     1     1 -8 1         1 -10 1         1

Integral Khovanov Homology (db, data source)

$\dim {\mathcal {G}}_{2r+i}\operatorname {KH} _{\mathbb {Z} }^{r}$ $i=-2$ $i=0$ $r=-4$ ${\mathbb {Z} }$ ${\mathbb {Z} }$ $r=-3$ $r=-2$ ${\mathbb {Z} }$ $r=-1$ ${\mathbb {Z} }_{2}$ ${\mathbb {Z} }$ $r=0$ ${\mathbb {Z} }$ ${\mathbb {Z} }$

Computer Talk

Much of the above data can be recomputed by Mathematica using the package KnotTheory`. See A Sample KnotTheory` Session.

Modifying This Page

Read me first: Modifying Knot Pages See/edit the Link Page master template (intermediate). See/edit the Link_Splice_Base (expert). Back to the top.

L2a1 L5a1