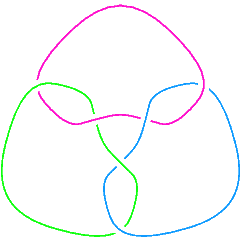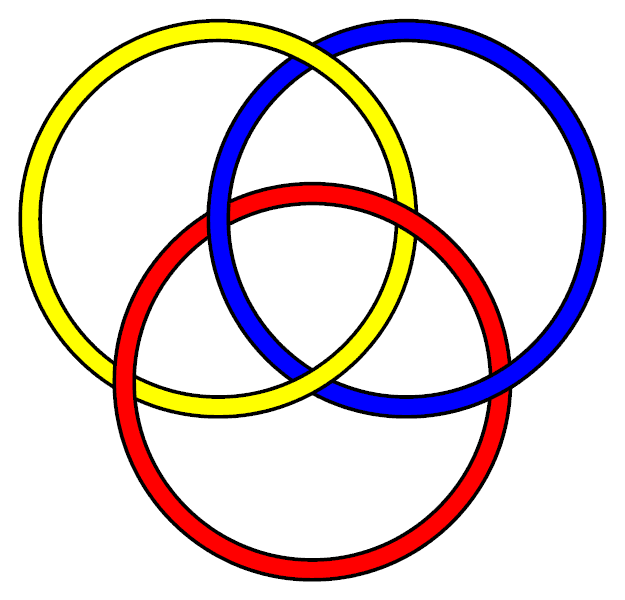L6a4: Difference between revisions
From Knot Atlas
Jump to navigationJump to search
DrorsRobot (talk | contribs) No edit summary |
m (Reverted edits by LielcOc4tn (Talk); changed back to last version by Drorbn) |
||
| (10 intermediate revisions by 8 users not shown) | |||
| Line 16: | Line 16: | ||
k = 4 | |
k = 4 | |
||
KnotilusURL = http://srankin.math.uwo.ca/cgi-bin/retrieve.cgi/1,-6,5,-3:4,-1,2,-5:6,-4,3,-2/goTop.html | |
KnotilusURL = http://srankin.math.uwo.ca/cgi-bin/retrieve.cgi/1,-6,5,-3:4,-1,2,-5:6,-4,3,-2/goTop.html | |
||
braid_table = <table cellspacing=0 cellpadding=0 border=0> |
braid_table = <table cellspacing=0 cellpadding=0 border=0 style="white-space: pre"> |
||
<tr><td>[[Image:BraidPart1.gif]][[Image:BraidPart0.gif]][[Image:BraidPart1.gif]][[Image:BraidPart0.gif]][[Image:BraidPart1.gif]][[Image:BraidPart0.gif]]</td></tr> |
<tr><td>[[Image:BraidPart1.gif]][[Image:BraidPart0.gif]][[Image:BraidPart1.gif]][[Image:BraidPart0.gif]][[Image:BraidPart1.gif]][[Image:BraidPart0.gif]]</td></tr> |
||
<tr><td>[[Image:BraidPart2.gif]][[Image:BraidPart3.gif]][[Image:BraidPart2.gif]][[Image:BraidPart3.gif]][[Image:BraidPart2.gif]][[Image:BraidPart3.gif]]</td></tr> |
<tr><td>[[Image:BraidPart2.gif]][[Image:BraidPart3.gif]][[Image:BraidPart2.gif]][[Image:BraidPart3.gif]][[Image:BraidPart2.gif]][[Image:BraidPart3.gif]]</td></tr> |
||
| Line 44: | Line 44: | ||
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
||
</tr> |
</tr> |
||
<tr valign=top><td colspan=2>Loading KnotTheory` (version of September |
<tr valign=top><td colspan=2>Loading KnotTheory` (version of September 3, 2005, 2:11:43)...</td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[2]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Crossings[Link[6, Alternating, 4]]</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[2]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Crossings[Link[6, Alternating, 4]]</nowiki></pre></td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[2]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>6</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[2]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>6</nowiki></pre></td></tr> |
||
Latest revision as of 23:07, 27 May 2009
|
|
|
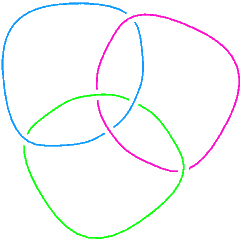 (Knotscape image) |
See the full Thistlethwaite Link Table (up to 11 crossings). |
|
The link L6a4 is in the Rolfsen table of links. It is also known as the "Borromean Link" or the "Borromean Rings". A Brunnian link - no two loops are linked directly together, but all three rings are collectively interlinked [9]. Visit Peter Cromwell's page on the Borromean Rings. |
 Medieval-style representation of the Borromean rings, used as an emblem of Lorenzo de Medici in San Pancrazio, Florence[1] |
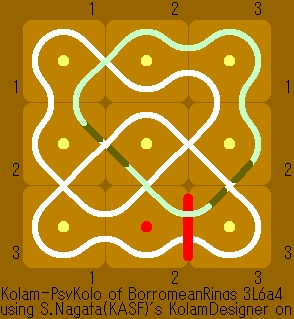 A kolam with 3 cycles [2] |
||
 The Colombo Mall in Lisboa [3] |
|||
 A "Borromean" bathroom tile (the Diane de Poitiers three interlaced crescents emblem) [4] |
 A Borromean link at the Fields Institute [5] |
||
 Borromean paper clips [6] |
 A Borromean link by Dylan Thurston [7] | ||
 A Borromean rattle by Sassy [8] |
Link Presentations
[edit Notes on L6a4's Link Presentations]
| Planar diagram presentation | X6172 X12,8,9,7 X4,12,1,11 X10,5,11,6 X8453 X2,9,3,10 |
| Gauss code | {1, -6, 5, -3}, {4, -1, 2, -5}, {6, -4, 3, -2} |
| A Braid Representative | ||||
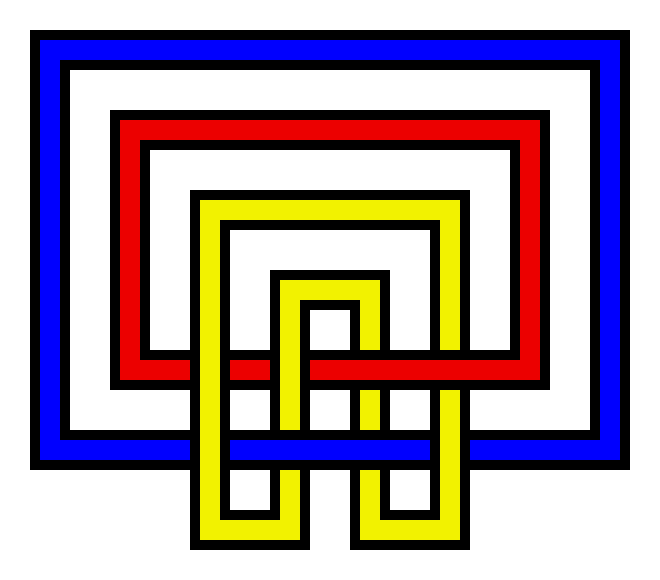
| A Morse Link Presentation | 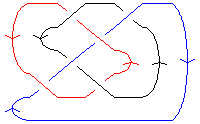
|
Polynomial invariants
| Multivariable Alexander Polynomial (in , , , ...) | (db) |
| Jones polynomial | (db) |
| Signature | 0 (db) |
| HOMFLY-PT polynomial | (db) |
| Kauffman polynomial | (db) |
Khovanov Homology
| The coefficients of the monomials are shown, along with their alternating sums (fixed , alternation over ). |
|
| Integral Khovanov Homology
(db, data source) |
|
Computer Talk
Much of the above data can be recomputed by Mathematica using the package KnotTheory`. See A Sample KnotTheory` Session.
Modifying This Page
| Read me first: Modifying Knot Pages
See/edit the Link Page master template (intermediate). See/edit the Link_Splice_Base (expert). Back to the top. |
|