A Sample KnotTheory` Session: Difference between revisions

From Knot Atlas
Jump to navigationJump to search
No edit summary
m (Reverted edits by TrocbOccch (Talk); changed back to last version by Drorbn)
 
(16 intermediate revisions by 4 users not shown)
Line 31: Line 31:
<!--END-->
<!--END-->


===[[Presentations]]===
===Presentations, Graphical Output and Tube Plots===

====[[Planar Diagrams]]====


<!--$$PD[K]$$-->
<!--$$PD[K]$$-->
Line 42: Line 44:
X[12, 5, 13, 6], X[4, 9, 5, 10], X[16, 12, 1, 11], X[10, 16, 11, 15]]</nowiki>}}
X[12, 5, 13, 6], X[4, 9, 5, 10], X[16, 12, 1, 11], X[10, 16, 11, 15]]</nowiki>}}
<!--END-->
<!--END-->

====[[Gauss Codes]]====


<!--$${GaussCode[K], GaussCode[L]}$$-->
<!--$${GaussCode[K], GaussCode[L]}$$-->
Line 54: Line 58:
{7, -1, -4, 5, 8, -2, -3, 6}]}</nowiki>}}
{7, -1, -4, 5, 8, -2, -3, 6}]}</nowiki>}}
<!--END-->
<!--END-->

====[[DT (Dowker-Thistlethwaite) Codes]]====


<!--$$DTCode[K]$$-->
<!--$$DTCode[K]$$-->
Line 62: Line 68:
out= <nowiki>DTCode[6, 8, 12, 14, 4, 16, 2, 10]</nowiki>}}
out= <nowiki>DTCode[6, 8, 12, 14, 4, 16, 2, 10]</nowiki>}}
<!--END-->
<!--END-->

====[[Braid Representatives]]====


<!--$$br = BR[K]$$-->
<!--$$br = BR[K]$$-->
Line 71: Line 79:
<!--END-->
<!--END-->


<!--$$Show[BraidPlot[br]]$$-->
<!--$${First[br], Crossings[br], BraidIndex[K]}$$-->
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{Graphics|
{{InOut|
n = 7 |
n = 7 |
in = <nowiki>Show[BraidPlot[br]]</nowiki> |
in = <nowiki>{First[br], Crossings[br], BraidIndex[K]}</nowiki> |
out= <nowiki>{3, 8, 3}</nowiki>}}
img= A_Sample_KnotTheory_Session_Out_7.gif |
out= <nowiki>-Graphics-</nowiki>}}
<!--END-->
<!--END-->


====[[Drawing Braids]]====
<!--$${First[br], Crossings[br], BraidIndex[K]}$$-->

<!--$$Show[BraidPlot[br]]$$-->
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{Graphics|
n = 8 |
n = 8 |
in = <nowiki>{First[br], Crossings[br], BraidIndex[K]}</nowiki> |
in = <nowiki>Show[BraidPlot[br]]</nowiki> |
img= A_Sample_KnotTheory_Session_Out_8.gif |
out= <nowiki>{3, 8, 3}</nowiki>}}
out= <nowiki>-Graphics-</nowiki>}}
<!--END-->
<!--END-->

====[[Drawing MorseLink Presentations]]====


<!--$$Show[DrawMorseLink[K]]$$-->
<!--$$Show[DrawMorseLink[K]]$$-->
Line 104: Line 116:
img= A_Sample_KnotTheory_Session_Out_10.gif |
img= A_Sample_KnotTheory_Session_Out_10.gif |
out= <nowiki>-Graphics-</nowiki>}}
out= <nowiki>-Graphics-</nowiki>}}
<!--END-->

====[[Drawing with TubePlot]]====

<!--$$Show[TubePlot[TK]]$$-->
<!--Robot Land, no human edits to "END"-->
{{Graphics|
n = 11 |
in = <nowiki>Show[TubePlot[TK]]</nowiki> |
img= A_Sample_KnotTheory_Session_Out_11.gif |
out= <nowiki>-Graphics3D-</nowiki>}}
<!--END-->
<!--END-->


Line 114: Line 137:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 11 |
n = 12 |
in = <nowiki>(#[K]&) /@ {
in = <nowiki>(#[K]&) /@ {
SymmetryType, UnknottingNumber, ThreeGenus,
SymmetryType, UnknottingNumber, ThreeGenus,
Line 129: Line 152:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 12 |
n = 13 |
in = <nowiki>alex = Alexander[K11][t]</nowiki> |
in = <nowiki>alex = Alexander[K11][t]</nowiki> |
out= <nowiki> -4 5 12 20 2 3 4
out= <nowiki> -4 5 12 20 2 3 4
Line 140: Line 163:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 13 |
n = 14 |
in = <nowiki>Conway[K11][t]</nowiki> |
in = <nowiki>Conway[K11][t]</nowiki> |
out= <nowiki> 2 4 6 8
out= <nowiki> 2 4 6 8
Line 151: Line 174:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 14 |
n = 15 |
in = <nowiki>Select[AllKnots[], (alex === Alexander[#][t])&]</nowiki> |
in = <nowiki>Select[AllKnots[], (alex === Alexander[#][t])&]</nowiki> |
out= <nowiki>{Knot[11, Alternating, 57], Knot[11, Alternating, 108],
out= <nowiki>{Knot[11, Alternating, 57], Knot[11, Alternating, 108],
Line 165: Line 188:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 15 |
n = 16 |
in = <nowiki>{KnotDet[K], KnotSignature[K]}</nowiki> |
in = <nowiki>{KnotDet[K], KnotSignature[K]}</nowiki> |
out= <nowiki>{37, 0}</nowiki>}}
out= <nowiki>{37, 0}</nowiki>}}
Line 175: Line 198:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 16 |
n = 17 |
in = <nowiki>J=Jones[K11][q]</nowiki> |
in = <nowiki>J=Jones[K11][q]</nowiki> |
out= <nowiki> -7 3 7 12 14 16 16 2 3 4
out= <nowiki> -7 3 7 12 14 16 16 2 3 4
Line 188: Line 211:
<!--Robot Land, no human edits to "END"-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
{{InOut|
n = 17 |
n = 18 |
in = <nowiki>Select[AllKnots[], (J === Jones[#][q] &#124;&#124; (J /. q -> 1/q) === Jones[#][q])&]</nowiki> |
in = <nowiki>Select[AllKnots[], (J === Jones[#][q] &#124;&#124; (J /. q -> 1/q) === Jones[#][q])&]</nowiki> |
out= <nowiki>{Knot[11, Alternating, 57], Knot[11, Alternating, 231]}</nowiki>}}
out= <nowiki>{Knot[11, Alternating, 57], Knot[11, Alternating, 231]}</nowiki>}}
Line 195: Line 218:
====[[The A2 Invariant]]====
====[[The A2 Invariant]]====


<!--$$A2Invariant[K11][q]$$-->
<!--$$A2Invariant[L][q]$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 19 |
in = <nowiki>A2Invariant[L][q]</nowiki> |
out= <nowiki> -30 3 4 5 4 3 2 -16 -14 -10 -8
q + --- + --- + --- + --- + --- + --- + q + q + q + q +
28 26 24 22 20 18
q q q q q q
-6
q</nowiki>}}
<!--END-->
<!--END-->


====[[The HOMFLY-PT Polynomial]]====
====[[The HOMFLY-PT Polynomial]]====


<!--$$HOMFLYPT[K11][q]$$-->
<!--$$HOMFLYPT[L][a, z]$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 20 |
in = <nowiki>HOMFLYPT[L][a, z]</nowiki> |
out= <nowiki> 6 8 10
4 8 a 2 a a 4 2 4 4
2 a - 2 a + -- - ---- + --- + 4 a z + a z
2 2 2
z z z</nowiki>}}
<!--END-->
<!--END-->


====[[The Kauffman Polynomial]]====
====[[The Kauffman Polynomial]]====


<!--$$Kauffman[K11][q]$$-->
<!--$$Kauffman[L][a, z]$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 21 |
in = <nowiki>Kauffman[L][a, z]</nowiki> |
out= <nowiki> 6 8 10 7 9
4 6 8 10 a 2 a a 2 a 2 a 7
2 a - 2 a - 9 a - 6 a + -- + ---- + --- - ---- - ---- + 8 a z +
2 2 2 z z
z z z
9 4 2 8 2 10 2 7 3 9 3 4 4
8 a z - 4 a z + 14 a z + 10 a z - 6 a z - 6 a z + a z -
8 4 10 4 7 5 9 5 8 6 10 6
7 a z - 6 a z + a z + a z + a z + a z</nowiki>}}
<!--END-->


===[[Finite Type (Vassiliev) Invariants]]===

<!--$${Vassiliev[2][K], Vassiliev[3][K]}$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 22 |
in = <nowiki>{Vassiliev[2][K], Vassiliev[3][K]}</nowiki> |
out= <nowiki>{-1, 0}</nowiki>}}
<!--END-->

===[[Khovanov Homology]]===

<!--$$Kh[TK][q, t]$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 23 |
in = <nowiki>Kh[TK][q, t]</nowiki> |
out= <nowiki> 23 25 27 2 31 3 29 4 31 4 33 5 35 5
q + q + q t + q t + q t + q t + q t + q t +
31 6 33 6 35 7 37 7 33 8 35 8 37 9
q t + q t + q t + q t + q t + 2 q t + q t +
39 9 37 10 41 11 39 12 41 12 45 12
2 q t + 2 q t + 3 q t + q t + 2 q t + q t +
43 13 45 13 43 14 47 14 47 15 47 16
2 q t + 2 q t + q t + q t + 2 q t + q t +
51 16 51 17
q t + q t</nowiki>}}
<!--END-->

===[[The Coloured Jones Polynomials]]===

<!--$$ColouredJones[K, #][q]& /@ {2, 3, 4, 5, 6, 7}$$-->
<!--Robot Land, no human edits to "END"-->
{{InOut|
n = 24 |
in = <nowiki>ColouredJones[K, #][q]& /@ {2, 3, 4, 5, 6, 7}</nowiki> |
out= <nowiki> -12 3 -10 9 14 3 28 25 14 47 29 25
{55 + q - --- + q + -- - -- - -- + -- - -- - -- + -- - -- - -- -
11 9 8 7 6 5 4 3 2 q
q q q q q q q q q
2 3 4 5 6 7 8 9
25 q - 29 q + 47 q - 14 q - 25 q + 28 q - 3 q - 14 q + 9 q +
10 11 12 -24 3 -22 5 -20 14
q - 3 q + q , 225 + q - --- + q + --- + q - --- -
23 21 19
q q q
6 29 17 43 40 55 73 64 108 61 146
--- + --- + --- - --- - --- + --- + --- - --- - --- + -- + --- -
18 17 16 15 14 13 12 11 10 9 8
q q q q q q q q q q q
53 177 38 205 26 216 6 2 3 4
-- - --- + -- + --- - -- - --- + - + 6 q - 216 q - 26 q + 205 q +
7 6 5 4 3 2 q
q q q q q q
5 6 7 8 9 10 11
38 q - 177 q - 53 q + 146 q + 61 q - 108 q - 64 q +
12 13 14 15 16 17 18
73 q + 55 q - 40 q - 43 q + 17 q + 29 q - 6 q -
19 20 21 22 23 24
14 q + q + 5 q + q - 3 q + q ,
-40 3 -38 5 3 -35 17 6 31
1233 + q - --- + q + --- - --- + q - --- + --- + --- +
39 37 36 34 33 32
q q q q q q
-31 82 16 96 69 52 216 146 120 216 260
q - --- - --- + --- + --- + --- - --- - --- + --- + --- + --- -
29 28 27 26 25 24 23 22 21 20
q q q q q q q q q q
323 393 7 340 605 292 631 265 347 945 149
--- - --- - --- + --- + --- - --- - --- - --- + --- + --- - --- -
19 18 17 16 15 14 13 12 11 10 9
q q q q q q q q q q q
759 522 261 1161 11 771 694 144 2
--- - --- + --- + ---- + -- - --- - --- + --- + 144 q - 694 q -
8 7 6 5 4 3 2 q
q q q q q q q
3 4 5 6 7 8 9
771 q + 11 q + 1161 q + 261 q - 522 q - 759 q - 149 q +
10 11 12 13 14 15
945 q + 347 q - 265 q - 631 q - 292 q + 605 q +
16 17 18 19 20 21 22
340 q - 7 q - 393 q - 323 q + 260 q + 216 q + 120 q -
23 24 25 26 27 28 29
146 q - 216 q + 52 q + 69 q + 96 q - 16 q - 82 q +
31 32 33 34 35 36 37 38 39
q + 31 q + 6 q - 17 q + q - 3 q + 5 q + q - 3 q +
40 -60 3 -58 5 3 3 2 5 8
q , 4841 + q - --- + q + --- - --- - --- - --- - --- + --- +
59 57 56 55 54 53 52
q q q q q q q
26 4 30 43 34 35 112 107 31 197 237
--- + --- - --- - --- - --- + --- + --- + --- - --- - --- - --- -
51 50 49 48 47 46 45 44 43 42 41
q q q q q q q q q q q
60 270 462 264 285 728 603 141 976 1094 186
--- + --- + --- + --- - --- - --- - --- + --- + --- + ---- + --- -
40 39 38 37 36 35 34 33 32 31 30
q q q q q q q q q q q
1134 1650 699 1099 2200 1387 888 2662 2125 494
---- - ---- - --- + ---- + ---- + ---- - --- - ---- - ---- + --- +
29 28 27 26 25 24 23 22 21 20
q q q q q q q q q q
2955 2877 9 3114 3506 568 3121 4033 1086 3040
---- + ---- + --- - ---- - ---- - --- + ---- + ---- + ---- - ---- -
19 18 17 16 15 14 13 12 11 10
q q q q q q q q q q
4387 1560 2881 4660 1920 2707 4762 2247 2479
---- - ---- + ---- + ---- + ---- - ---- - ---- - ---- + ---- +
9 8 7 6 5 4 3 2 q
q q q q q q q q
2 3 4 5 6 7
2479 q - 2247 q - 4762 q - 2707 q + 1920 q + 4660 q + 2881 q -
8 9 10 11 12 13
1560 q - 4387 q - 3040 q + 1086 q + 4033 q + 3121 q -
14 15 16 17 18 19
568 q - 3506 q - 3114 q + 9 q + 2877 q + 2955 q +
20 21 22 23 24 25
494 q - 2125 q - 2662 q - 888 q + 1387 q + 2200 q +
26 27 28 29 30 31
1099 q - 699 q - 1650 q - 1134 q + 186 q + 1094 q +
32 33 34 35 36 37
976 q + 141 q - 603 q - 728 q - 285 q + 264 q +
38 39 40 41 42 43 44
462 q + 270 q - 60 q - 237 q - 197 q - 31 q + 107 q +
45 46 47 48 49 50 51
112 q + 35 q - 34 q - 43 q - 30 q + 4 q + 26 q +
52 53 54 55 56 57 58 59 60
8 q - 5 q - 2 q - 3 q - 3 q + 5 q + q - 3 q + q ,
-84 3 -82 5 3 3 6 10 3 3
26111 + q - --- + q + --- - --- - --- - --- + --- - --- + --- +
83 81 80 79 78 77 76 75
q q q q q q q q
29 15 31 49 14 16 61 153 14 117 273
--- - --- - --- - --- + --- + --- + --- + --- + --- - --- - --- -
74 73 72 71 70 69 68 67 66 65 64
q q q q q q q q q q q
149 92 203 641 463 57 691 870 1005 189 1343
--- - --- + --- + --- + --- + --- - --- - --- - ---- - --- + ---- +
63 62 61 60 59 58 57 56 55 54 53
q q q q q q q q q q q
1882 1543 247 1725 3355 2544 658 3470 4894 2812
---- + ---- - --- - ---- - ---- - ---- + --- + ---- + ---- + ---- -
52 51 50 49 48 47 46 45 44 43
q q q q q q q q q q
590 5842 7188 3328 2689 8288 8456 4312 5674
--- - ---- - ---- - ---- + ---- + ---- + ---- + ---- - ---- -
42 41 40 39 38 37 36 35 34
q q q q q q q q q
11801 10070 1954 8878 14013 11845 1776 13643 16608
----- - ----- - ---- + ---- + ----- + ----- - ---- - ----- - ----- -
33 32 31 30 29 28 27 26 25
q q q q q q q q q
8872 6032 16953 18906 4000 12400 20628 15121 1645
---- + ---- + ----- + ----- + ---- - ----- - ----- - ----- + ---- +
24 23 22 21 20 19 18 17 16
q q q q q q q q q
17146 23466 9075 9763 22071 19122 2210 15962 25565
----- + ----- + ---- - ---- - ----- - ----- - ---- + ----- + ----- +
15 14 13 12 11 10 9 8 7
q q q q q q q q q
12389 7226 22014 21134 4957 14414 2
----- - ---- - ----- - ----- - ---- + ----- + 14414 q - 4957 q -
6 5 4 3 2 q
q q q q q
3 4 5 6 7 8
21134 q - 22014 q - 7226 q + 12389 q + 25565 q + 15962 q -
9 10 11 12 13 14
2210 q - 19122 q - 22071 q - 9763 q + 9075 q + 23466 q +
15 16 17 18 19
17146 q + 1645 q - 15121 q - 20628 q - 12400 q +
20 21 22 23 24 25
4000 q + 18906 q + 16953 q + 6032 q - 8872 q - 16608 q -
26 27 28 29 30 31
13643 q - 1776 q + 11845 q + 14013 q + 8878 q - 1954 q -
32 33 34 35 36 37
10070 q - 11801 q - 5674 q + 4312 q + 8456 q + 8288 q +
38 39 40 41 42 43
2689 q - 3328 q - 7188 q - 5842 q - 590 q + 2812 q +
44 45 46 47 48 49
4894 q + 3470 q + 658 q - 2544 q - 3355 q - 1725 q -
50 51 52 53 54 55
247 q + 1543 q + 1882 q + 1343 q - 189 q - 1005 q -
56 57 58 59 60 61 62
870 q - 691 q + 57 q + 463 q + 641 q + 203 q - 92 q -
63 64 65 66 67 68 69
149 q - 273 q - 117 q + 14 q + 153 q + 61 q + 16 q +
70 71 72 73 74 75 76
14 q - 49 q - 31 q - 15 q + 29 q + 3 q - 3 q +
77 78 79 80 81 82 83 84
10 q - 6 q - 3 q - 3 q + 5 q + q - 3 q + q ,
-112 3 -110 5 3 3 6 6
127145 + q - ---- + q + ---- - ---- - ---- - ---- + ---- +
111 109 108 107 106 105
q q q q q q
12 8 6 10 16 26 41 7 74 44
---- - ---- + ---- + ---- - ---- - --- - --- + --- + --- + --- +
104 103 102 101 100 99 98 97 96 95
q q q q q q q q q q
71 43 78 159 283 154 143 317 550 516 93
--- + --- - --- - --- - --- - --- + --- + --- + --- + --- + --- -
94 93 92 91 90 89 88 87 86 85 84
q q q q q q q q q q q
417 1159 1332 683 256 1725 2573 2216 836 1934
--- - ---- - ---- - --- + --- + ---- + ---- + ---- + --- - ---- -
83 82 81 80 79 78 77 76 75 74
q q q q q q q q q q
4278 4774 3301 913 5542 8189 7815 2389 5364
---- - ---- - ---- + --- + ---- + ---- + ---- + ---- - ---- -
73 72 71 70 69 68 67 66 65
q q q q q q q q q
11792 14143 8598 2327 13890 21402 18063 4775 12979
----- - ----- - ---- + ---- + ----- + ----- + ----- + ---- - ----- -
64 63 62 61 60 59 58 57 56
q q q q q q q q q
28137 29695 16138 7462 32099 41960 31319 3221
----- - ----- - ----- + ---- + ----- + ----- + ----- + ---- -
55 54 53 52 51 50 49 48
q q q q q q q q
31814 52797 48414 18546 26269 60101 65456 37209
----- - ----- - ----- - ----- + ----- + ----- + ----- + ----- -
47 46 45 44 43 42 41 40
q q q q q q q q
15734 62982 80416 56819 1416 61028 91744 75657
----- - ----- - ----- - ----- + ---- + ----- + ----- + ----- +
39 38 37 36 35 34 33 32
q q q q q q q q
14955 55290 98994 91866 31357 46837 102372 104758
----- - ----- - ----- - ----- - ----- + ----- + ------ + ------ +
31 30 29 28 27 26 25 24
q q q q q q q q
46339 37376 102713 113992 58991 28020 101063 120209
----- - ----- - ------ - ------ - ----- + ----- + ------ + ------ +
23 22 21 20 19 18 17 16
q q q q q q q q
68876 19792 98300 123826 76313 12722 95254 125943
----- - ----- - ----- - ------ - ----- + ----- + ----- + ------ +
15 14 13 12 11 10 9 8
q q q q q q q q
81699 7156 92151 126720 85773 2177 89089
----- - ---- - ----- - ------ - ----- + ---- + ----- + 89089 q +
7 6 5 4 3 2 q
q q q q q q
2 3 4 5 6 7
2177 q - 85773 q - 126720 q - 92151 q - 7156 q + 81699 q +
8 9 10 11 12
125943 q + 95254 q + 12722 q - 76313 q - 123826 q -
13 14 15 16 17
98300 q - 19792 q + 68876 q + 120209 q + 101063 q +
18 19 20 21 22
28020 q - 58991 q - 113992 q - 102713 q - 37376 q +
23 24 25 26 27
46339 q + 104758 q + 102372 q + 46837 q - 31357 q -
28 29 30 31 32
91866 q - 98994 q - 55290 q + 14955 q + 75657 q +
33 34 35 36 37
91744 q + 61028 q + 1416 q - 56819 q - 80416 q -
38 39 40 41 42
62982 q - 15734 q + 37209 q + 65456 q + 60101 q +
43 44 45 46 47
26269 q - 18546 q - 48414 q - 52797 q - 31814 q +
48 49 50 51 52
3221 q + 31319 q + 41960 q + 32099 q + 7462 q -
53 54 55 56 57
16138 q - 29695 q - 28137 q - 12979 q + 4775 q +
58 59 60 61 62
18063 q + 21402 q + 13890 q + 2327 q - 8598 q -
63 64 65 66 67 68
14143 q - 11792 q - 5364 q + 2389 q + 7815 q + 8189 q +
69 70 71 72 73 74
5542 q + 913 q - 3301 q - 4774 q - 4278 q - 1934 q +
75 76 77 78 79 80
836 q + 2216 q + 2573 q + 1725 q + 256 q - 683 q -
81 82 83 84 85 86
1332 q - 1159 q - 417 q + 93 q + 516 q + 550 q +
87 88 89 90 91 92 93
317 q + 143 q - 154 q - 283 q - 159 q - 78 q + 43 q +
94 95 96 97 98 99 100
71 q + 44 q + 74 q + 7 q - 41 q - 26 q - 16 q +
101 102 103 104 105 106 107
10 q + 6 q - 8 q + 12 q + 6 q - 6 q - 3 q -
108 109 110 111 112
3 q + 5 q + q - 3 q + q }</nowiki>}}
<!--END-->
<!--END-->

Latest revision as of 16:23, 27 May 2009


KnotTheory`/Navigation 

The Mathematica Package KnotTheory`

Setup

The first step is to load KnotTheory` as in the Setup section:

In[1]:= << KnotTheory`

Loading KnotTheory` (version of September 14, 2005, 13:37:36)...

8 17.gif
8_17 		K11a231.gif
K11a231
L8n6.gif
L8n6 		T(7,5).jpg
T(7,5)

Let us now introduce the four star knots that will accompany us throughout this session:

In[2]:= K = Knot[8, 17]; K11 = Knot[11, Alternating, 231]; L = Link[8, NonAlternating, 6]; TK = TorusKnot[7,5];

Presentations, Graphical Output and Tube Plots

Planar Diagrams

In[3]:= PD[K]
Out[3]= PD[X[6, 2, 7, 1], X[14, 8, 15, 7], X[8, 3, 9, 4], X[2, 13, 3, 14], X[12, 5, 13, 6], X[4, 9, 5, 10], X[16, 12, 1, 11], X[10, 16, 11, 15]]

Gauss Codes

In[4]:= {GaussCode[K], GaussCode[L]}
Out[4]= {GaussCode[1, -4, 3, -6, 5, -1, 2, -3, 6, -8, 7, -5, 4, -2, 8, -7], GaussCode[{1, -7, 2, -8}, {-5, 4, -6, 3}, {7, -1, -4, 5, 8, -2, -3, 6}]}

DT (Dowker-Thistlethwaite) Codes

In[5]:= DTCode[K]
Out[5]= DTCode[6, 8, 12, 14, 4, 16, 2, 10]

Braid Representatives

In[6]:= br = BR[K]
Out[6]= BR[3, {-1, -1, 2, -1, 2, -1, 2, 2}]
In[7]:= {First[br], Crossings[br], BraidIndex[K]}
Out[7]= {3, 8, 3}

Drawing Braids

In[8]:= Show[BraidPlot[br]]
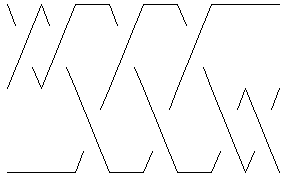
A Sample KnotTheory Session Out 8.gif
Out[8]= -Graphics-

Drawing MorseLink Presentations

In[9]:= Show[DrawMorseLink[K]]
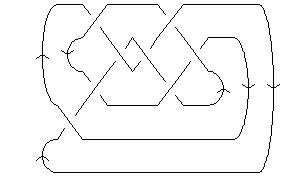
A Sample KnotTheory Session Out 9.gif
Out[9]= -Graphics-
In[10]:= Show[DrawMorseLink[L]]
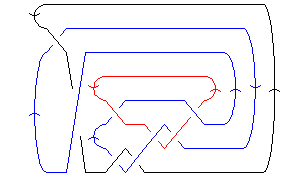
A Sample KnotTheory Session Out 10.gif
Out[10]= -Graphics-

Drawing with TubePlot

In[11]:= Show[TubePlot[TK]]
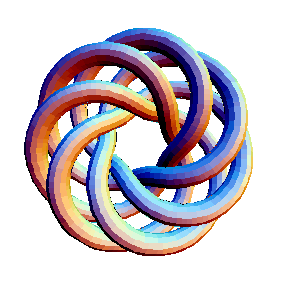
A Sample KnotTheory Session Out 11.gif
Out[11]= -Graphics3D-

Three Dimensional Invariants

In[12]:= (#[K]&) /@ { SymmetryType, UnknottingNumber, ThreeGenus, BridgeIndex, SuperBridgeIndex, NakanishiIndex }
Out[12]= {NegativeAmphicheiral, 1, 3, 3, 4, 1}

Polynomial Invariants

The Alexander-Conway Polynomial

In[13]:= alex = Alexander[K11][t]
Out[13]= -4 5 12 20 2 3 4 -23 - t + -- - -- + -- + 20 t - 12 t + 5 t - t 3 2 t t t
In[14]:= Conway[K11][t]
Out[14]= 2 4 6 8 1 + t - 2 t - 3 t - t
"Similar" Knots (within the Atlas)
In[15]:= Select[AllKnots[], (alex === Alexander[#][t])&]
Out[15]= {Knot[11, Alternating, 57], Knot[11, Alternating, 108], Knot[11, Alternating, 139], Knot[11, Alternating, 231]}
K11a57.gif
K11a57 		K11a108.gif
K11a108 		K11a139.gif
K11a139 		K11a231.gif
K11a231
The Determinant and the Signature
In[16]:= {KnotDet[K], KnotSignature[K]}
Out[16]= {37, 0}

The Jones Polynomial

In[17]:= J=Jones[K11][q]
Out[17]= -7 3 7 12 14 16 16 2 3 4 -12 + q - -- + -- - -- + -- - -- + -- + 10 q - 5 q + 2 q - q 6 5 4 3 2 q q q q q q
"Similar" Knots (within the Atlas)
In[18]:= Select[AllKnots[], (J === Jones[#][q] || (J /. q -> 1/q) === Jones[#][q])&]
Out[18]= {Knot[11, Alternating, 57], Knot[11, Alternating, 231]}

The A2 Invariant

In[19]:= A2Invariant[L][q]
Out[19]= -30 3 4 5 4 3 2 -16 -14 -10 -8 q + --- + --- + --- + --- + --- + --- + q + q + q + q + 28 26 24 22 20 18 q q q q q q -6 q

The HOMFLY-PT Polynomial

In[20]:= HOMFLYPT[L][a, z]
Out[20]= 6 8 10 4 8 a 2 a a 4 2 4 4 2 a - 2 a + -- - ---- + --- + 4 a z + a z 2 2 2 z z z

The Kauffman Polynomial

In[21]:= Kauffman[L][a, z]
Out[21]= 6 8 10 7 9 4 6 8 10 a 2 a a 2 a 2 a 7 2 a - 2 a - 9 a - 6 a + -- + ---- + --- - ---- - ---- + 8 a z + 2 2 2 z z z z z 9 4 2 8 2 10 2 7 3 9 3 4 4 8 a z - 4 a z + 14 a z + 10 a z - 6 a z - 6 a z + a z - 8 4 10 4 7 5 9 5 8 6 10 6 7 a z - 6 a z + a z + a z + a z + a z


Finite Type (Vassiliev) Invariants

In[22]:= {Vassiliev[2][K], Vassiliev[3][K]}
Out[22]= {-1, 0}

Khovanov Homology

In[23]:= Kh[TK][q, t]
Out[23]= 23 25 27 2 31 3 29 4 31 4 33 5 35 5 q + q + q t + q t + q t + q t + q t + q t + 31 6 33 6 35 7 37 7 33 8 35 8 37 9 q t + q t + q t + q t + q t + 2 q t + q t + 39 9 37 10 41 11 39 12 41 12 45 12 2 q t + 2 q t + 3 q t + q t + 2 q t + q t + 43 13 45 13 43 14 47 14 47 15 47 16 2 q t + 2 q t + q t + q t + 2 q t + q t + 51 16 51 17 q t + q t

The Coloured Jones Polynomials

In[24]:= ColouredJones[K, #][q]& /@ {2, 3, 4, 5, 6, 7}
Out[24]= -12 3 -10 9 14 3 28 25 14 47 29 25 {55 + q - --- + q + -- - -- - -- + -- - -- - -- + -- - -- - -- - 11 9 8 7 6 5 4 3 2 q q q q q q q q q q 2 3 4 5 6 7 8 9 25 q - 29 q + 47 q - 14 q - 25 q + 28 q - 3 q - 14 q + 9 q + 10 11 12 -24 3 -22 5 -20 14 q - 3 q + q , 225 + q - --- + q + --- + q - --- - 23 21 19 q q q 6 29 17 43 40 55 73 64 108 61 146 --- + --- + --- - --- - --- + --- + --- - --- - --- + -- + --- - 18 17 16 15 14 13 12 11 10 9 8 q q q q q q q q q q q 53 177 38 205 26 216 6 2 3 4 -- - --- + -- + --- - -- - --- + - + 6 q - 216 q - 26 q + 205 q + 7 6 5 4 3 2 q q q q q q q 5 6 7 8 9 10 11 38 q - 177 q - 53 q + 146 q + 61 q - 108 q - 64 q + 12 13 14 15 16 17 18 73 q + 55 q - 40 q - 43 q + 17 q + 29 q - 6 q - 19 20 21 22 23 24 14 q + q + 5 q + q - 3 q + q , -40 3 -38 5 3 -35 17 6 31 1233 + q - --- + q + --- - --- + q - --- + --- + --- + 39 37 36 34 33 32 q q q q q q -31 82 16 96 69 52 216 146 120 216 260 q - --- - --- + --- + --- + --- - --- - --- + --- + --- + --- - 29 28 27 26 25 24 23 22 21 20 q q q q q q q q q q 323 393 7 340 605 292 631 265 347 945 149 --- - --- - --- + --- + --- - --- - --- - --- + --- + --- - --- - 19 18 17 16 15 14 13 12 11 10 9 q q q q q q q q q q q 759 522 261 1161 11 771 694 144 2 --- - --- + --- + ---- + -- - --- - --- + --- + 144 q - 694 q - 8 7 6 5 4 3 2 q q q q q q q q 3 4 5 6 7 8 9 771 q + 11 q + 1161 q + 261 q - 522 q - 759 q - 149 q + 10 11 12 13 14 15 945 q + 347 q - 265 q - 631 q - 292 q + 605 q + 16 17 18 19 20 21 22 340 q - 7 q - 393 q - 323 q + 260 q + 216 q + 120 q - 23 24 25 26 27 28 29 146 q - 216 q + 52 q + 69 q + 96 q - 16 q - 82 q + 31 32 33 34 35 36 37 38 39 q + 31 q + 6 q - 17 q + q - 3 q + 5 q + q - 3 q + 40 -60 3 -58 5 3 3 2 5 8 q , 4841 + q - --- + q + --- - --- - --- - --- - --- + --- + 59 57 56 55 54 53 52 q q q q q q q 26 4 30 43 34 35 112 107 31 197 237 --- + --- - --- - --- - --- + --- + --- + --- - --- - --- - --- - 51 50 49 48 47 46 45 44 43 42 41 q q q q q q q q q q q 60 270 462 264 285 728 603 141 976 1094 186 --- + --- + --- + --- - --- - --- - --- + --- + --- + ---- + --- - 40 39 38 37 36 35 34 33 32 31 30 q q q q q q q q q q q 1134 1650 699 1099 2200 1387 888 2662 2125 494 ---- - ---- - --- + ---- + ---- + ---- - --- - ---- - ---- + --- + 29 28 27 26 25 24 23 22 21 20 q q q q q q q q q q 2955 2877 9 3114 3506 568 3121 4033 1086 3040 ---- + ---- + --- - ---- - ---- - --- + ---- + ---- + ---- - ---- - 19 18 17 16 15 14 13 12 11 10 q q q q q q q q q q 4387 1560 2881 4660 1920 2707 4762 2247 2479 ---- - ---- + ---- + ---- + ---- - ---- - ---- - ---- + ---- + 9 8 7 6 5 4 3 2 q q q q q q q q q 2 3 4 5 6 7 2479 q - 2247 q - 4762 q - 2707 q + 1920 q + 4660 q + 2881 q - 8 9 10 11 12 13 1560 q - 4387 q - 3040 q + 1086 q + 4033 q + 3121 q - 14 15 16 17 18 19 568 q - 3506 q - 3114 q + 9 q + 2877 q + 2955 q + 20 21 22 23 24 25 494 q - 2125 q - 2662 q - 888 q + 1387 q + 2200 q + 26 27 28 29 30 31 1099 q - 699 q - 1650 q - 1134 q + 186 q + 1094 q + 32 33 34 35 36 37 976 q + 141 q - 603 q - 728 q - 285 q + 264 q + 38 39 40 41 42 43 44 462 q + 270 q - 60 q - 237 q - 197 q - 31 q + 107 q + 45 46 47 48 49 50 51 112 q + 35 q - 34 q - 43 q - 30 q + 4 q + 26 q + 52 53 54 55 56 57 58 59 60 8 q - 5 q - 2 q - 3 q - 3 q + 5 q + q - 3 q + q , -84 3 -82 5 3 3 6 10 3 3 26111 + q - --- + q + --- - --- - --- - --- + --- - --- + --- + 83 81 80 79 78 77 76 75 q q q q q q q q 29 15 31 49 14 16 61 153 14 117 273 --- - --- - --- - --- + --- + --- + --- + --- + --- - --- - --- - 74 73 72 71 70 69 68 67 66 65 64 q q q q q q q q q q q 149 92 203 641 463 57 691 870 1005 189 1343 --- - --- + --- + --- + --- + --- - --- - --- - ---- - --- + ---- + 63 62 61 60 59 58 57 56 55 54 53 q q q q q q q q q q q 1882 1543 247 1725 3355 2544 658 3470 4894 2812 ---- + ---- - --- - ---- - ---- - ---- + --- + ---- + ---- + ---- - 52 51 50 49 48 47 46 45 44 43 q q q q q q q q q q 590 5842 7188 3328 2689 8288 8456 4312 5674 --- - ---- - ---- - ---- + ---- + ---- + ---- + ---- - ---- - 42 41 40 39 38 37 36 35 34 q q q q q q q q q 11801 10070 1954 8878 14013 11845 1776 13643 16608 ----- - ----- - ---- + ---- + ----- + ----- - ---- - ----- - ----- - 33 32 31 30 29 28 27 26 25 q q q q q q q q q 8872 6032 16953 18906 4000 12400 20628 15121 1645 ---- + ---- + ----- + ----- + ---- - ----- - ----- - ----- + ---- + 24 23 22 21 20 19 18 17 16 q q q q q q q q q 17146 23466 9075 9763 22071 19122 2210 15962 25565 ----- + ----- + ---- - ---- - ----- - ----- - ---- + ----- + ----- + 15 14 13 12 11 10 9 8 7 q q q q q q q q q 12389 7226 22014 21134 4957 14414 2 ----- - ---- - ----- - ----- - ---- + ----- + 14414 q - 4957 q - 6 5 4 3 2 q q q q q q 3 4 5 6 7 8 21134 q - 22014 q - 7226 q + 12389 q + 25565 q + 15962 q - 9 10 11 12 13 14 2210 q - 19122 q - 22071 q - 9763 q + 9075 q + 23466 q + 15 16 17 18 19 17146 q + 1645 q - 15121 q - 20628 q - 12400 q + 20 21 22 23 24 25 4000 q + 18906 q + 16953 q + 6032 q - 8872 q - 16608 q - 26 27 28 29 30 31 13643 q - 1776 q + 11845 q + 14013 q + 8878 q - 1954 q - 32 33 34 35 36 37 10070 q - 11801 q - 5674 q + 4312 q + 8456 q + 8288 q + 38 39 40 41 42 43 2689 q - 3328 q - 7188 q - 5842 q - 590 q + 2812 q + 44 45 46 47 48 49 4894 q + 3470 q + 658 q - 2544 q - 3355 q - 1725 q - 50 51 52 53 54 55 247 q + 1543 q + 1882 q + 1343 q - 189 q - 1005 q - 56 57 58 59 60 61 62 870 q - 691 q + 57 q + 463 q + 641 q + 203 q - 92 q - 63 64 65 66 67 68 69 149 q - 273 q - 117 q + 14 q + 153 q + 61 q + 16 q + 70 71 72 73 74 75 76 14 q - 49 q - 31 q - 15 q + 29 q + 3 q - 3 q + 77 78 79 80 81 82 83 84 10 q - 6 q - 3 q - 3 q + 5 q + q - 3 q + q , -112 3 -110 5 3 3 6 6 127145 + q - ---- + q + ---- - ---- - ---- - ---- + ---- + 111 109 108 107 106 105 q q q q q q 12 8 6 10 16 26 41 7 74 44 ---- - ---- + ---- + ---- - ---- - --- - --- + --- + --- + --- + 104 103 102 101 100 99 98 97 96 95 q q q q q q q q q q 71 43 78 159 283 154 143 317 550 516 93 --- + --- - --- - --- - --- - --- + --- + --- + --- + --- + --- - 94 93 92 91 90 89 88 87 86 85 84 q q q q q q q q q q q 417 1159 1332 683 256 1725 2573 2216 836 1934 --- - ---- - ---- - --- + --- + ---- + ---- + ---- + --- - ---- - 83 82 81 80 79 78 77 76 75 74 q q q q q q q q q q 4278 4774 3301 913 5542 8189 7815 2389 5364 ---- - ---- - ---- + --- + ---- + ---- + ---- + ---- - ---- - 73 72 71 70 69 68 67 66 65 q q q q q q q q q 11792 14143 8598 2327 13890 21402 18063 4775 12979 ----- - ----- - ---- + ---- + ----- + ----- + ----- + ---- - ----- - 64 63 62 61 60 59 58 57 56 q q q q q q q q q 28137 29695 16138 7462 32099 41960 31319 3221 ----- - ----- - ----- + ---- + ----- + ----- + ----- + ---- - 55 54 53 52 51 50 49 48 q q q q q q q q 31814 52797 48414 18546 26269 60101 65456 37209 ----- - ----- - ----- - ----- + ----- + ----- + ----- + ----- - 47 46 45 44 43 42 41 40 q q q q q q q q 15734 62982 80416 56819 1416 61028 91744 75657 ----- - ----- - ----- - ----- + ---- + ----- + ----- + ----- + 39 38 37 36 35 34 33 32 q q q q q q q q 14955 55290 98994 91866 31357 46837 102372 104758 ----- - ----- - ----- - ----- - ----- + ----- + ------ + ------ + 31 30 29 28 27 26 25 24 q q q q q q q q 46339 37376 102713 113992 58991 28020 101063 120209 ----- - ----- - ------ - ------ - ----- + ----- + ------ + ------ + 23 22 21 20 19 18 17 16 q q q q q q q q 68876 19792 98300 123826 76313 12722 95254 125943 ----- - ----- - ----- - ------ - ----- + ----- + ----- + ------ + 15 14 13 12 11 10 9 8 q q q q q q q q 81699 7156 92151 126720 85773 2177 89089 ----- - ---- - ----- - ------ - ----- + ---- + ----- + 89089 q + 7 6 5 4 3 2 q q q q q q q 2 3 4 5 6 7 2177 q - 85773 q - 126720 q - 92151 q - 7156 q + 81699 q + 8 9 10 11 12 125943 q + 95254 q + 12722 q - 76313 q - 123826 q - 13 14 15 16 17 98300 q - 19792 q + 68876 q + 120209 q + 101063 q + 18 19 20 21 22 28020 q - 58991 q - 113992 q - 102713 q - 37376 q + 23 24 25 26 27 46339 q + 104758 q + 102372 q + 46837 q - 31357 q - 28 29 30 31 32 91866 q - 98994 q - 55290 q + 14955 q + 75657 q + 33 34 35 36 37 91744 q + 61028 q + 1416 q - 56819 q - 80416 q - 38 39 40 41 42 62982 q - 15734 q + 37209 q + 65456 q + 60101 q + 43 44 45 46 47 26269 q - 18546 q - 48414 q - 52797 q - 31814 q + 48 49 50 51 52 3221 q + 31319 q + 41960 q + 32099 q + 7462 q - 53 54 55 56 57 16138 q - 29695 q - 28137 q - 12979 q + 4775 q + 58 59 60 61 62 18063 q + 21402 q + 13890 q + 2327 q - 8598 q - 63 64 65 66 67 68 14143 q - 11792 q - 5364 q + 2389 q + 7815 q + 8189 q + 69 70 71 72 73 74 5542 q + 913 q - 3301 q - 4774 q - 4278 q - 1934 q + 75 76 77 78 79 80 836 q + 2216 q + 2573 q + 1725 q + 256 q - 683 q - 81 82 83 84 85 86 1332 q - 1159 q - 417 q + 93 q + 516 q + 550 q + 87 88 89 90 91 92 93 317 q + 143 q - 154 q - 283 q - 159 q - 78 q + 43 q + 94 95 96 97 98 99 100 71 q + 44 q + 74 q + 7 q - 41 q - 26 q - 16 q + 101 102 103 104 105 106 107 10 q + 6 q - 8 q + 12 q + 6 q - 6 q - 3 q - 108 109 110 111 112 3 q + 5 q + q - 3 q + q }
Retrieved from "https://katlas.org/index.php?title=A_Sample_KnotTheory%60_Session&oldid=1693863"