A Sample KnotTheory` Session

KnotTheory`/Navigation  The Mathematica Package KnotTheory` Acknowledgement Setup Naming and Enumeration Presentations Planar Diagrams Some further details Gauss Codes DT (Dowker-Thistlethwaite) Codes Braid Representatives MorseLink Presentations Arc Presentations Conway Notation Graphical Input Graphical Output Drawing Planar Diagrams How does it work? Drawing MorseLink Presentations Drawing Braids Structure and Operations Invariants Invariants from Braid Theory Three Dimensional Invariants The Alexander-Conway Polynomial The Multivariable Alexander Polynomial The Determinant and the Signature The Jones Polynomial How is the Jones polynomial computed? The Coloured Jones Polynomials The A2 Invariant Quantum knot invariants The HOMFLY-PT Polynomial The Kauffman Polynomial Finite Type (Vassiliev) Invariants Khovanov Homology Heegaard Floer Knot Homology R-Matrix Invariants Extras Included with KnotTheory` Drawing with TubePlot Standalone_TubePlot Using the LinKnot package WikiLink QuantumGroups` Lightly Documented Features A Sample KnotTheory` Session Further Usage Example Prime Links with a Non-Prime Component "Rubberband" Brunnian Links Identifying Knots within a List Cabling Burau's Theorem Further Knot Theory Software KnotPlot Knotscape Xknots Extending/Modifying KnotTheory` Bugs How to Edit this Manual...

Setup

The first step is to load KnotTheory` as in the Setup section:

In[1]:= << KnotTheory`

Loading KnotTheory` (version of September 14, 2005, 13:37:36)...

8_17

K11a231

L8n6

T(7,5)

Let us now introduce the four star knots that will accompany us throughout this session:

In[2]:=

K = Knot[8, 17]; K11 = Knot[11, Alternating, 231]; L = Link[8, NonAlternating, 6]; TK = TorusKnot[7,5];

Presentations

In[3]:=	PD[K]
Out[3]=	PD[X[6, 2, 7, 1], X[14, 8, 15, 7], X[8, 3, 9, 4], X[2, 13, 3, 14], X[12, 5, 13, 6], X[4, 9, 5, 10], X[16, 12, 1, 11], X[10, 16, 11, 15]]

In[4]:=	{GaussCode[K], GaussCode[L]}
Out[4]=	{GaussCode[1, -4, 3, -6, 5, -1, 2, -3, 6, -8, 7, -5, 4, -2, 8, -7], GaussCode[{1, -7, 2, -8}, {-5, 4, -6, 3}, {7, -1, -4, 5, 8, -2, -3, 6}]}

In[5]:=	DTCode[K]
Out[5]=	DTCode[6, 8, 12, 14, 4, 16, 2, 10]

In[6]:=	br = BR[K]
Out[6]=	BR[3, {-1, -1, 2, -1, 2, -1, 2, 2}]

In[7]:=	Show[BraidPlot[br]]
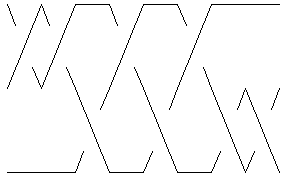
Out[7]=	-Graphics-

In[8]:=	{First[br], Crossings[br], BraidIndex[K]}
Out[8]=	{3, 8, 3}

In[9]:=	Show[DrawMorseLink[K]]
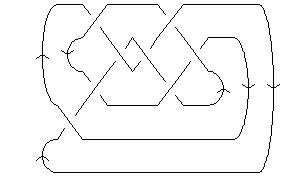
Out[9]=	-Graphics-

In[10]:=	Show[DrawMorseLink[L]]
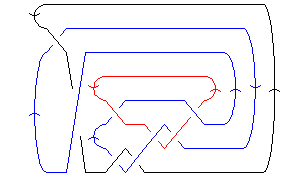
Out[10]=	-Graphics-

Three Dimensional Invariants

In[11]:=	(#[K]&) /@ { SymmetryType, UnknottingNumber, ThreeGenus, BridgeIndex, SuperBridgeIndex, NakanishiIndex }
Out[11]=	{NegativeAmphicheiral, 1, 3, 3, 4, 1}