Heegaard Floer Knot Homology: Difference between revisions
No edit summary |
No edit summary |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
{{Manual TOC Sidebar}} |
{{Manual TOC Sidebar}} |
||
In 2007, [http://www.math.unizh.ch/user/jdroz/ Jean-Marie Droz] of the University of Zurich (working along with [http://www.math.unizh.ch/index.php?id=1819&no_cache=1&key1=578&no_cache=1 Anna Beliakova]) wrote a Python program to compute the (hat-version) Heegaard-Floer Knot Homology <math>\widehat{\operatorname{HFK}}(K)</math> of a knot <math>K</math>. His program is integrated into <code>KnotTheory`</code>, though to run it, you must have [http://python.org/ Python] as well as the Python library [http://psyco.sourceforge.net/ |
In 2007, [http://www.math.unizh.ch/user/jdroz/ Jean-Marie Droz] of the University of Zurich (working along with [http://www.math.unizh.ch/index.php?id=1819&no_cache=1&key1=578&no_cache=1 Anna Beliakova]) wrote a Python program to compute the (hat-version) Heegaard-Floer Knot Homology <math>\widehat{\operatorname{HFK}}(K)</math> of a knot <math>K</math> (see {{arXiv|0803.2379}}). His program is integrated into <code>KnotTheory`</code>, though to run it, you must have [http://python.org/ Python] as well as the Python library [http://psyco.sourceforge.net/ Psyco] installed on your system. |
||
{{Startup Note}} |
{{Startup Note}} |
||
| Line 104: | Line 104: | ||
<!--END--> |
<!--END--> |
||
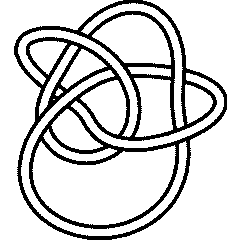
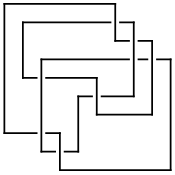
On July 6, 2006, [[User:AnonMoos]] [[User_talk:Drorbn#Here.27s_one|asked]] [[User:Drorbn]] if he could identify the knot in the left hand side picture below. At the time it was impossible using the tools available with <code>KnotTheory`</code> (though of course, it |
On July 6, 2006, [[User:AnonMoos]] [[User_talk:Drorbn#Here.27s_one|asked]] [[User:Drorbn]] if he could identify the knot in the left hand side picture below. At the time it was impossible using the tools available with <code>KnotTheory`</code> - using any of many invariants, the answer can be found to be either the mirror of [[K11n34]] or the mirror of [[K11n42]], but <code>KnotTheory`</code> couldn't tell which one it is (though of course, it is possible to do it "by hand"). The 2007 addition <math>\widehat{\operatorname{HFK}}</math> does the job, though. Indeed, we first extract the mystery knot's [[DT (Dowker-Thistlethwaite) Codes|DT (Dowker-Thistlethwaite) Code]] using the picture on the right hand side below, then compute <math>\widehat{\operatorname{HFK}}</math>, and then search for it within the <math>\widehat{\operatorname{HFK}}</math>'s of all knots with up to 11 crossings: |
||
{| align=center width=80% |
{| align=center width=80% |
||
Latest revision as of 11:40, 20 March 2008
In 2007, Jean-Marie Droz of the University of Zurich (working along with Anna Beliakova) wrote a Python program to compute the (hat-version) Heegaard-Floer Knot Homology of a knot (see arXiv:0803.2379). His program is integrated into KnotTheory`, though to run it, you must have Python as well as the Python library Psyco installed on your system.
(For In[1] see Setup)
|
| ||||||||
The Heegaard-Floer Knot Homology is a categorification of the Alexander polynomial. Let us test that for the knot 8_19:
In[3]:=
|
hfk = HFKHat[K = Knot[8, 19]][t, m]
|
Out[3]=
|
2 -3 m 5 2 6 3
m + t + -- + m t + m t
2
t
|
In[4]:=
|
{hfk /. m -> -1, Alexander[K][t]}
|
Out[4]=
|
-3 -2 2 3 -3 -2 2 3
{1 + t - t - t + t , 1 + t - t - t + t }
|
The knot 8_19 is the first knot in the Rolfsen Knot Table whose Heegaard-Floer Knot Homology is not "diagonal". Let us test that. The homology is "on diagonal", iff its Poincare polynomial, evaluated at , is a monomial:
In[5]:=
|
Select[AllKnots[{3, 8}], (Head[HFKHat[#][t, 1/t]] == Plus) &]
|
Out[5]=
|
{Knot[8, 19]}
|
In[6]:=
|
hfk /. m -> 1/t
|
Out[6]=
|
4 -2
-- + t
3
t
|
 K11n34 |
 K11n42 |
The (mirrored) Conway knot K11n34 and the (mirrored) Kinoshita-Terasaka knot K11n42 are a mutant pair, and are notoriously difficult to tell apart. Let us check that an array of standard knot polynomials fails to separate them, yet succeeds:
In[7]:=
|
K1 = Knot["K11n34"]; K2 = Knot["K11n42"];
test[invt_] := (invt[K1] =!= invt[K2]);
test /@ {
Alexander, MultivariableAlexander, Jones, HOMFLYPT, Kauffman, Kh, HFKHat
}
|
Out[7]=
|
{False, False, False, False, False, False, True}
|
Indeed,
In[8]:=
|
{HFKHat[K1][t, m], HFKHat[K2][t, m]}
|
Out[8]=
|
2 1 1 3 3 3 3
{3 + - + ----- + ----- + ----- + ----- + ---- + --- + 3 t + 3 m t +
m 4 3 3 3 3 2 2 2 2 m t
m t m t m t m t m t
2 2 2 2 3 3 3
3 m t + 3 m t + m t + m t ,
6 1 1 4 4 2 2 2
7 + - + ----- + ----- + ---- + --- + 4 t + 4 m t + m t + m t }
m 3 2 2 2 2 m t
m t m t m t
|
On July 6, 2006, User:AnonMoos asked User:Drorbn if he could identify the knot in the left hand side picture below. At the time it was impossible using the tools available with KnotTheory` - using any of many invariants, the answer can be found to be either the mirror of K11n34 or the mirror of K11n42, but KnotTheory` couldn't tell which one it is (though of course, it is possible to do it "by hand"). The 2007 addition does the job, though. Indeed, we first extract the mystery knot's DT (Dowker-Thistlethwaite) Code using the picture on the right hand side below, then compute , and then search for it within the 's of all knots with up to 11 crossings:

|

|
In[9]:=
|
K3 = DTCode[6, 8, 14, 12, 4, -18, 2, -20, -22, -10, -16];
|
In[10]:=
|
H = HFKHat[Mirror[K3]][t, m]
|
Out[10]=
|
2 1 1 3 3 3 3
3 + - + ----- + ----- + ----- + ----- + ---- + --- + 3 t + 3 m t +
m 4 3 3 3 3 2 2 2 2 m t
m t m t m t m t m t
2 2 2 2 3 3 3
3 m t + 3 m t + m t + m t
|
In[11]:=
|
Select[AllKnots[], HFKHat[#][t, m] == H &]
|
Out[11]=
|
{Knot[11, NonAlternating, 34]}
|
And so the mystery knot is the Conway knot, the mirror of K11n34.