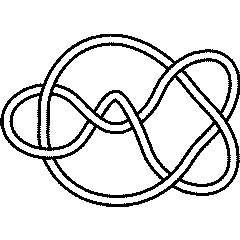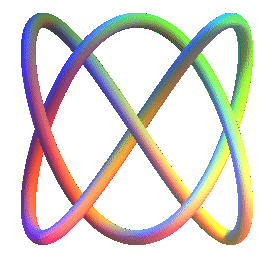7 4: Difference between revisions
No edit summary |
DrorsRobot (talk | contribs) No edit summary |
||
| Line 1: | Line 1: | ||
<!-- WARNING! WARNING! WARNING! |
|||
<!-- This page was |
<!-- This page was generated from the splice template [[Rolfsen_Splice_Base]]. Please do not edit! |
||
<!-- --> <!-- |
|||
<!-- You probably want to edit the template referred to immediately below. (See [[Category:Knot Page Template]].) |
|||
--> |
|||
<!-- This page itself was created by running [[Media:KnotPageSpliceRobot.nb]] on [[Rolfsen_Splice_Base]]. --> |
|||
<!-- <math>\text{Null}</math> --> |
|||
<!-- <math>\text{Null}</math> --> |
|||
{{Rolfsen Knot Page| |
{{Rolfsen Knot Page| |
||
n = 7 | |
n = 7 | |
||
| Line 47: | Line 50: | ||
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
<td align=left><pre style="color: red; border: 0px; padding: 0em"><< KnotTheory`</pre></td> |
||
</tr> |
</tr> |
||
<tr valign=top><td colspan=2>Loading KnotTheory` (version of August 29, 2005, 15: |
<tr valign=top><td colspan=2>Loading KnotTheory` (version of August 29, 2005, 15:27:48)...</td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[2]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>PD[Knot[7, 4]]</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[2]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>PD[Knot[7, 4]]</nowiki></pre></td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[2]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>PD[X[6, 2, 7, 1], X[12, 6, 13, 5], X[14, 8, 1, 7], X[8, 14, 9, 13], |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[2]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>PD[X[6, 2, 7, 1], X[12, 6, 13, 5], X[14, 8, 1, 7], X[8, 14, 9, 13], |
||
| Line 63: | Line 66: | ||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[7]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>4</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[7]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>4</nowiki></pre></td></tr> |
||
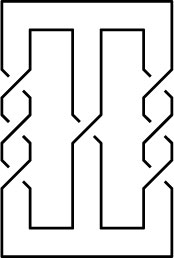
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[8]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Show[DrawMorseLink[Knot[7, 4]]]</nowiki></pre></td></tr><tr><td></td><td align=left>[[Image:7_4_ML.gif]]</td></tr><tr valign=top><td><tt><font color=blue>Out[8]=</font></tt><td><tt><font color=black>-Graphics-</font></tt></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[8]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>Show[DrawMorseLink[Knot[7, 4]]]</nowiki></pre></td></tr><tr><td></td><td align=left>[[Image:7_4_ML.gif]]</td></tr><tr valign=top><td><tt><font color=blue>Out[8]=</font></tt><td><tt><font color=black>-Graphics-</font></tt></td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[9]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>(#[Knot[7, 4]]&) /@ { |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[9]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki> (#[Knot[7, 4]]&) /@ { |
||
SymmetryType, UnknottingNumber, ThreeGenus, |
|||
BridgeIndex, SuperBridgeIndex, NakanishiIndex |
|||
}</nowiki></pre></td></tr> |
|||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[9]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>{Reversible, 2, 1, 2, {3, 4}, 1}</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>Out[9]= </nowiki></pre></td><td><pre style="color: black; border: 0px; padding: 0em"><nowiki>{Reversible, 2, 1, 2, {3, 4}, 1}</nowiki></pre></td></tr> |
||
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[10]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>alex = Alexander[Knot[7, 4]][t]</nowiki></pre></td></tr> |
<tr valign=top><td><pre style="color: blue; border: 0px; padding: 0em"><nowiki>In[10]:=</nowiki></pre></td><td><pre style="color: red; border: 0px; padding: 0em"><nowiki>alex = Alexander[Knot[7, 4]][t]</nowiki></pre></td></tr> |
||
Revision as of 17:44, 31 August 2005
|
|
|
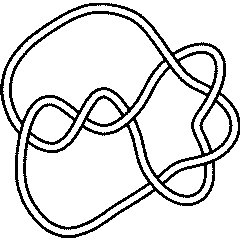
 (KnotPlot image) |
See the full Rolfsen Knot Table. Visit 7 4's page at the Knot Server (KnotPlot driven, includes 3D interactive images!) |
|
Simplest version of Endless knot symbol. |
 a knot seen at the Castle of Kornik [3] |
 A 7-4 knot reduced from TakaraMusubi with 9 crossings [4] |
 TakaraMusubi knot seen in Japanese symbols, or Kolam in South India [5] |
|
 A laser cut by Tom Longtin [6] |
|||
|
Knot presentations
| Planar diagram presentation | X6271 X12,6,13,5 X14,8,1,7 X8,14,9,13 X2,12,3,11 X10,4,11,3 X4,10,5,9 |
| Gauss code | 1, -5, 6, -7, 2, -1, 3, -4, 7, -6, 5, -2, 4, -3 |
| Dowker-Thistlethwaite code | 6 10 12 14 4 2 8 |
| Conway Notation | [313] |
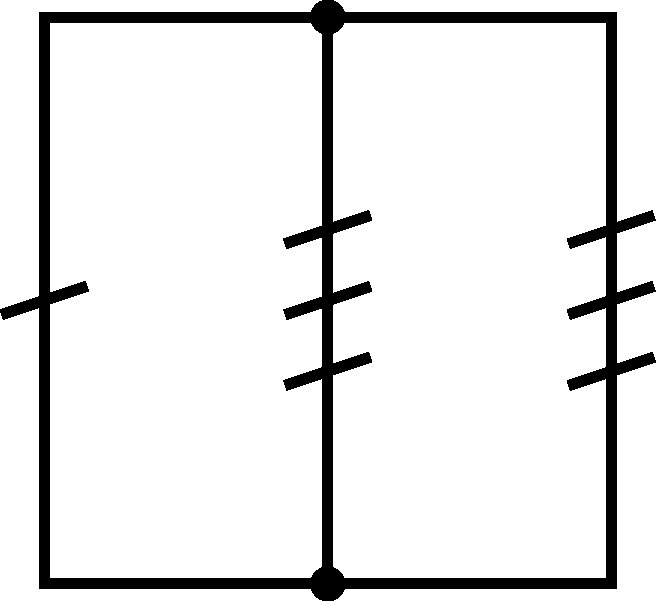
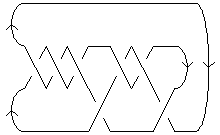
| Minimum Braid Representative | A Morse Link Presentation | An Arc Presentation | ||||
Length is 9, width is 4, Braid index is 4 |
|
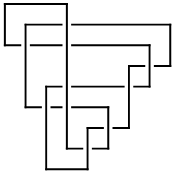 [{3, 5}, {6, 4}, {5, 7}, {2, 6}, {8, 3}, {7, 9}, {1, 8}, {9, 2}, {4, 1}] |
[edit Notes on presentations of 7 4]
KnotTheory`. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of May 31, 2006, 14:15:20.091.
|
In[3]:=
|
K = Knot["7 4"];
|
In[4]:=
|
PD[K]
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
Out[4]=
|
X6271 X12,6,13,5 X14,8,1,7 X8,14,9,13 X2,12,3,11 X10,4,11,3 X4,10,5,9 |
In[5]:=
|
GaussCode[K]
|
Out[5]=
|
1, -5, 6, -7, 2, -1, 3, -4, 7, -6, 5, -2, 4, -3 |
In[6]:=
|
DTCode[K]
|
Out[6]=
|
6 10 12 14 4 2 8 |
(The path below may be different on your system)
In[7]:=
|
AppendTo[$Path, "C:/bin/LinKnot/"];
|
In[8]:=
|
ConwayNotation[K]
|
Out[8]=
|
[313] |
In[9]:=
|
br = BR[K]
|
KnotTheory::credits: The minimum braids representing the knots with up to 10 crossings were provided by Thomas Gittings. See arXiv:math.GT/0401051.
|
Out[9]=
|
In[10]:=
|
{First[br], Crossings[br], BraidIndex[K]}
|
KnotTheory::credits: The braid index data known to KnotTheory` is taken from Charles Livingston's http://www.indiana.edu/~knotinfo/.
|
KnotTheory::loading: Loading precomputed data in IndianaData`.
|
Out[10]=
|
{ 4, 9, 4 } |
In[11]:=
|
Show[BraidPlot[br]]
|
Out[11]=
|
-Graphics- |
In[12]:=
|
Show[DrawMorseLink[K]]
|
KnotTheory::credits: "MorseLink was added to KnotTheory` by Siddarth Sankaran at the University of Toronto in the summer of 2005."
|
KnotTheory::credits: "DrawMorseLink was written by Siddarth Sankaran at the University of Toronto in the summer of 2005."
|

|
Out[12]=
|
-Graphics- |
In[13]:=
|
ap = ArcPresentation[K]
|
Out[13]=
|
ArcPresentation[{3, 5}, {6, 4}, {5, 7}, {2, 6}, {8, 3}, {7, 9}, {1, 8}, {9, 2}, {4, 1}] |
In[14]:=
|
Draw[ap]
|

|
Out[14]=
|
-Graphics- |
Three dimensional invariants
|
Four dimensional invariants
|
Polynomial invariants
A1 Invariants.
| Weight | Invariant |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 |
A2 Invariants.
| Weight | Invariant |
|---|---|
| 1,0 | |
| 1,1 | |
| 2,0 | |
| 3,0 |
A3 Invariants.
| Weight | Invariant |
|---|---|
| 0,1,0 | |
| 1,0,0 |
A4 Invariants.
| Weight | Invariant |
|---|---|
| 0,1,0,0 | |
| 1,0,0,0 |
B2 Invariants.
| Weight | Invariant |
|---|---|
| 0,1 | |
| 1,0 |
D4 Invariants.
| Weight | Invariant |
|---|---|
| 1,0,0,0 |
G2 Invariants.
| Weight | Invariant |
|---|---|
| 1,0 |
.
KnotTheory`, as shown in the (simulated) Mathematica session below. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting. This Mathematica session is also available (albeit only for the knot 5_2) as the notebook PolynomialInvariantsSession.nb.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of August 31, 2006, 11:25:27.5625.
|
In[3]:=
|
K = Knot["7 4"];
|
In[4]:=
|
Alexander[K][t]
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
Out[4]=
|
In[5]:=
|
Conway[K][z]
|
Out[5]=
|
In[6]:=
|
Alexander[K, 2][t]
|
KnotTheory::credits: The program Alexander[K, r] to compute Alexander ideals was written by Jana Archibald at the University of Toronto in the summer of 2005.
|
Out[6]=
|
In[7]:=
|
{KnotDet[K], KnotSignature[K]}
|
Out[7]=
|
{ 15, 2 } |
In[8]:=
|
Jones[K][q]
|
KnotTheory::loading: Loading precomputed data in Jones4Knots`.
|
Out[8]=
|
In[9]:=
|
HOMFLYPT[K][a, z]
|
KnotTheory::credits: The HOMFLYPT program was written by Scott Morrison.
|
Out[9]=
|
In[10]:=
|
Kauffman[K][a, z]
|
KnotTheory::loading: Loading precomputed data in Kauffman4Knots`.
|
Out[10]=
|
"Similar" Knots (within the Atlas)
Same Alexander/Conway Polynomial: {9_2,}
Same Jones Polynomial (up to mirroring, ): {}
KnotTheory`. Your input (in red) is realistic; all else should have the same content as in a real mathematica session, but with different formatting.
(The path below may be different on your system, and possibly also the KnotTheory` date)
In[1]:=
|
AppendTo[$Path, "C:/drorbn/projects/KAtlas/"];
<< KnotTheory`
|
Loading KnotTheory` version of May 31, 2006, 14:15:20.091.
|
In[3]:=
|
K = Knot["7 4"];
|
In[4]:=
|
{A = Alexander[K][t], J = Jones[K][q]}
|
KnotTheory::loading: Loading precomputed data in PD4Knots`.
|
KnotTheory::loading: Loading precomputed data in Jones4Knots`.
|
Out[4]=
|
{ , } |
In[5]:=
|
DeleteCases[Select[AllKnots[], (A === Alexander[#][t]) &], K]
|
KnotTheory::loading: Loading precomputed data in DTCode4KnotsTo11`.
|
KnotTheory::credits: The GaussCode to PD conversion was written by Siddarth Sankaran at the University of Toronto in the summer of 2005.
|
Out[5]=
|
{9_2,} |
In[6]:=
|
DeleteCases[
Select[
AllKnots[],
(J === Jones[#][q] || (J /. q -> 1/q) === Jones[#][q]) &
],
K
]
|
KnotTheory::loading: Loading precomputed data in Jones4Knots11`.
|
Out[6]=
|
{} |
Vassiliev invariants
| V2 and V3: | (4, 8) |
| V2,1 through V6,9: |
|
V2,1 through V6,9 were provided by Petr Dunin-Barkowski <barkovs@itep.ru>, Andrey Smirnov <asmirnov@itep.ru>, and Alexei Sleptsov <sleptsov@itep.ru> and uploaded on October 2010 by User:Drorbn. Note that they are normalized differently than V2 and V3.
Khovanov Homology
| The coefficients of the monomials are shown, along with their alternating sums (fixed , alternation over ). The squares with yellow highlighting are those on the "critical diagonals", where or , where 2 is the signature of 7 4. Nonzero entries off the critical diagonals (if any exist) are highlighted in red. |
|
| Integral Khovanov Homology
(db, data source) |
|
The Coloured Jones Polynomials
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 |
Computer Talk
Much of the above data can be recomputed by Mathematica using the package KnotTheory`. See A Sample KnotTheory` Session, or any of the Computer Talk sections above.
Modifying This Page
| Read me first: Modifying Knot Pages
See/edit the Rolfsen Knot Page master template (intermediate). See/edit the Rolfsen_Splice_Base (expert). Back to the top. |
|